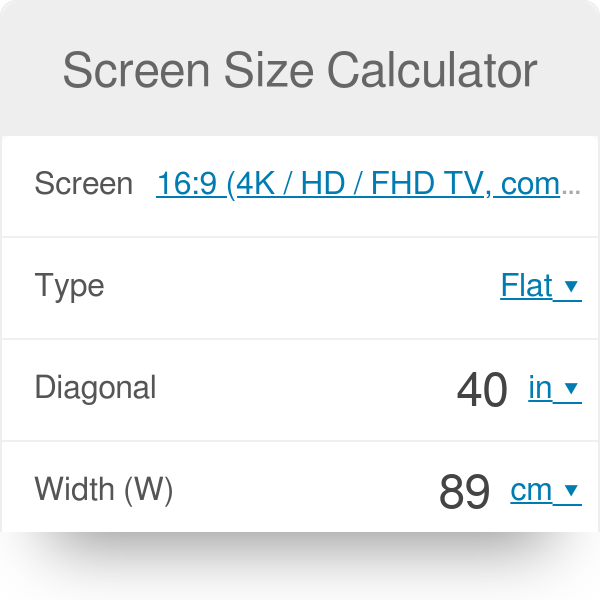
lcd panel size calculator free sample

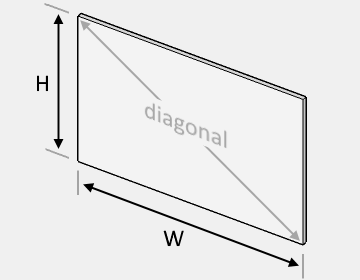
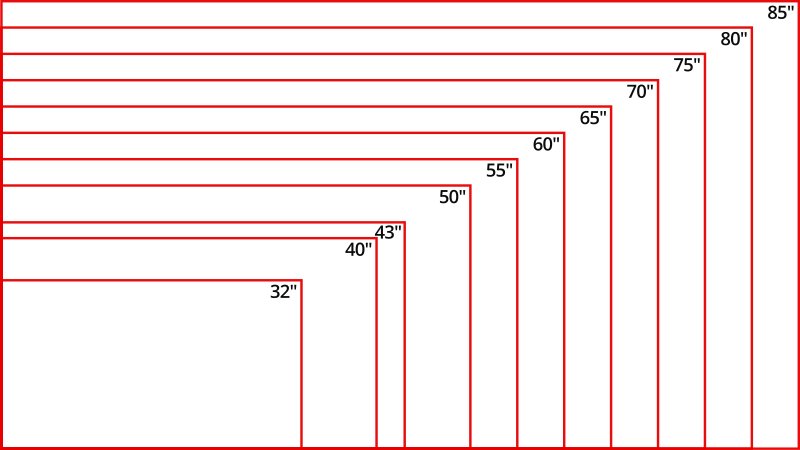
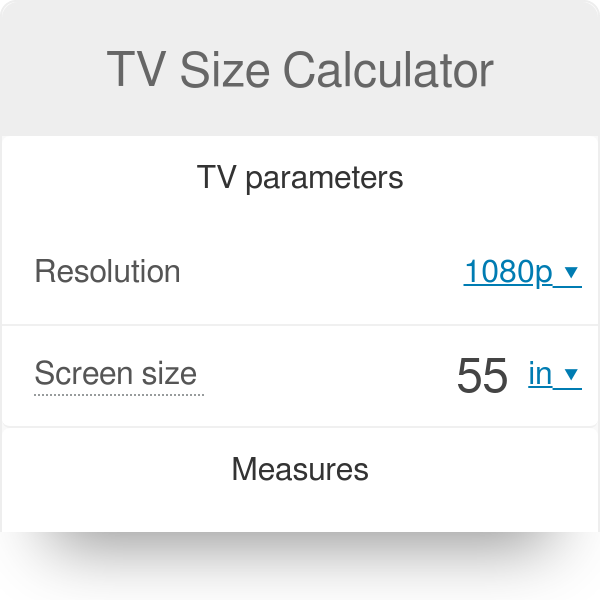
The screen size calculator can help you determine the screen dimensions. Have you ever wondered what the statement, the diagonal of the screen is equal to 40" means? How much space does it take up on the wall? What are its height and width? Here you can determine the monitor size or phone screen dimensions. If you"re curious enough, you can even determine how to measure a curved TV screen size!

If you want to find the ideal size of your TV or its distance from your eyes, check out this TV size calculator. Another useful tool is this great projector calculator, which can help in fulfilling your dreams about a home cinema.

Repeat the steps for iPhone 8 Plus, choosing a 16:9 aspect ratio and 5.5-inch diagonal. And what do you get? 4.8" for screen width, 2.7" height, and 12.93 in² screen size. So even though the first iPhone has a longer diagonal, the screen size is smaller than in iPhone 8 plus. (iPhone X also has the big cutout and the curved edges, but we are not taking them into account - even with that simplification, the screen area is smaller).

Our screen size calculator offers the possibility to estimate both a flat and curved screen size. As we"ve already learned how to proceed with a flat monitor, let"s see the differences for a curved one.

How do I measure a curved TV screen size, then? - you may ask. To evaluate the screen"s length, height, and area, we can follow the same equations as for a flat one:

Assuming the screen"s length is the arc length of a circle, we can find the base depth and width by using the following formulas which we use in the screen size calculator:

If you"re after the size of the screen"s diagonal, you can either measure it or use Pythagoras" theorem where a & b are the screen"s length and width.

The size of a 16:9 screen depends on how long the screen"s diagonal is, as 16:9 is merely the ratio of the screen"s width to its height. If you have the screens diagonal, you can multiply that measurement by 0.872 to get the screen"s width. You can also multiply the diagonal by 0.49 to get the screen"s height. Then, if you wish to find the screen area, multiply the width by the height.

Sample size calculators can be used for product surveys, marketing surveys, satisfaction surveys, school surveys, employee surveys, and more. They can help to assess overall satisfaction in your workplace, get more insight on your target market, and receive feedback.

A lot goes into determining the best viewing distance, and there are several different criteria you can use. Aside from size, things like resolution and even how strong your eyesight is can affect how you see the screen. Because everyone"s eyesight is different, this is less an exact science and more of a general guide based on scientific principles of vision and resolution.

Our size and distance tool above is based on the 30° guideline that is suitable for mixed usage, but you can find distances for a variety of sizes at 40° here.Learn more about the human visual field.

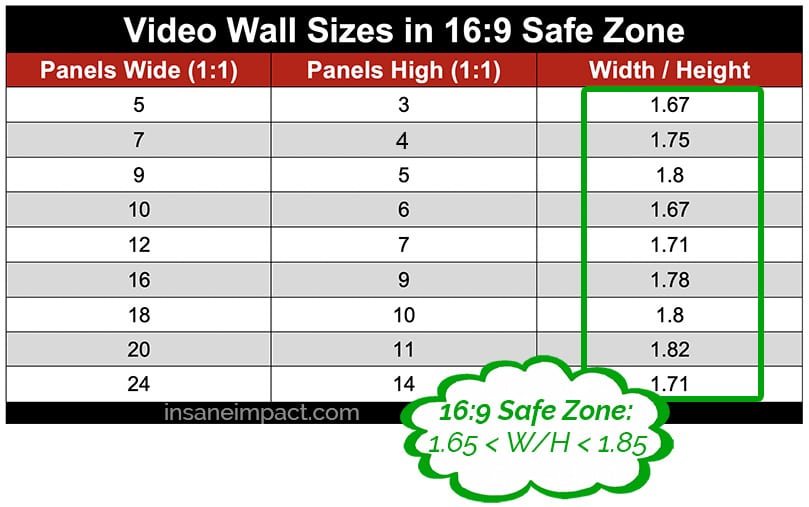
This chart shows the point at which an upgrade in resolution becomes worth it depending on size and distance to the TV. Each line represents the optimal viewing distance for each resolution, but any TV that falls within the range of that color will be suitable to notice a difference in picture quality. So, for example, if you have a 65 inch TV, the viewing distance at which the eye can actually process the details of 4k content is about 4 feet. However, any distance between 4 and about 8.5 feet will be enough to appreciate the difference between 4k and 1080p on a 65 inch TV. Go too far, and the image will look identical to 1080p HD.

The chart suggests that at a certain point, 4k UHD may not be worth the upgrade—if you"re sitting more than 7 feet away and have a 55" TV, for instance. Really, though, this chart is just a guide, and as 4k TVs have become the standard, the question of whether it"s worth it or not is a moot point. While your eyes may not be able to tell the difference at a certain point, your next TV will more than likely be a 4k TV anyway. Knowing the optimal viewing distance for the resolution can help you determine a living room setup that takes full advantage of your TV"s resolution, but since angular resolution is almost a non-issue with UHD content, we recommend using our calculator tool at the top of the page, which is based on the optimal field of vision.

You"re probably thinking something along the lines of "My couch is 10" away from my TV, which according to the chart means I need a 75 inch TV. This is insane!" It"s true that if you want to take full advantage of higher resolutions, that"s the ideal size you should get. That said, this may not be possible for everyone, which brings us to budget.

The price of a TV is usually exponential to its size. Size isn"t the only factor though, as resolution, panel type, and features all play into it as well. Looking at 65 inch TVs, for instance, an OLED like the LG CX OLED is inevitably going to cost more than a budget LED TV like the Hisense H8G, and both of these will seem downright cheap compared to an 8k TV like the Samsung Q900TS 8k QLED. Fortunately, though, as technology improves and the availability of higher resolution TVs expands, larger TVs have become more common and therefore more affordable. Feel free to compare the prices of our picks for the best 65 inch TVs, the best 70 to 75 inch TVs, and the best 80 to 85 inch TVs to really see the difference that size makes.

We recommend a field of vision of about 30 degrees for mixed usage. In general, we also recommend getting a 4k TV since lower resolution TVs are becoming harder to find. To easily find out what size you should buy, you can divide your TV viewing distance (in inches) by 1.6 (or use our TV size calculator above) which roughly equals a 30-degree angle. If the best size is outside your budget, just get the biggest TV you can afford. These are guidelines, after all, and since most TVs nowadays are 4k, you can"t really go wrong with the size that works for you, especially since picture quality also depends a lot on the content and viewing conditions. Ideally, you would optimize the capacity of your TV by getting one that"s large enough for you to notice all the visual detail that 4k has to offer, but ultimately, you should watch however feels most comfortable to you, whatever the size and distance may be.

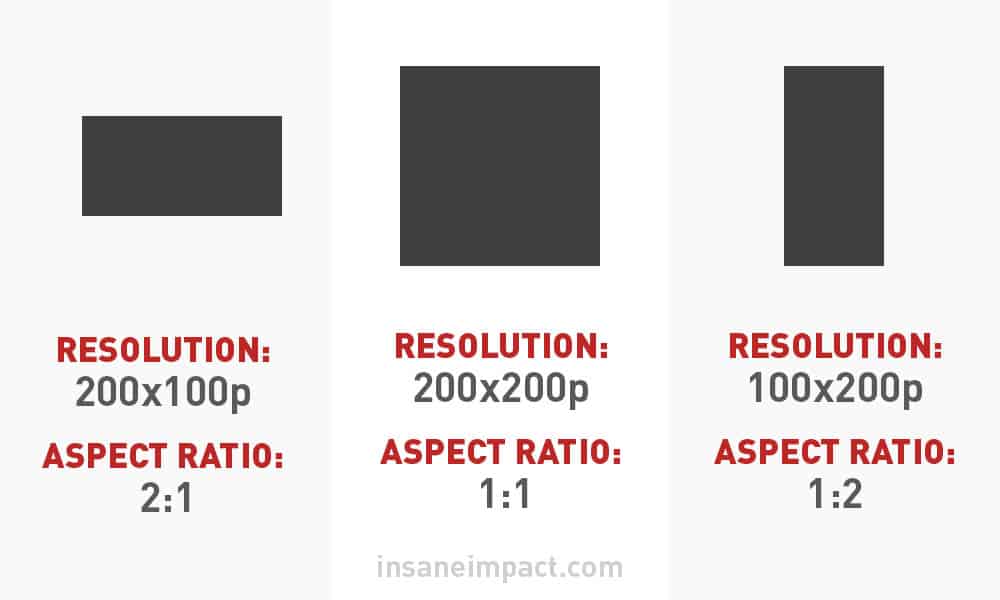
To achieve a perfect aspect ratio, you must employ the use of an aspect ratio calculator. It reduces any margin of error. Check the what is aspect ratio post.

Calculate international shoe sizes with the shoe size converter. When shopping for shoes in Europe you might have noticed the different shoe sizing system.

The shoe sizing guide can help you understand and measure your feet correct. You can also download and use the printable shoe size chart to accurately check your shoe size.

Sample size calculators can be used for product surveys, marketing surveys, satisfaction surveys, school surveys, employee surveys, and more. They can help to assess overall satisfaction in your workplace, get more insight on your target market, and receive feedback.

This basic online calculator is similar to a small handheld calculator and has the standard four functions for addition, subtraction, division and multiplication. Like most 4-function calculators it also includes keys for percent, square, square root and pi. This basic calculator has decimal precision up to 10 digits and offers these functions:

Zoom: Increase the size of the calculator in your browser using your browser"s zoom feature. The size of the calculator, text and buttons changes proportionately.

Touch Screen Zoom: Increase the size of the calculator on your touch screen by zooming with your fingers. The size of the calculator, text and buttons changes proportionately.

Text Size: In some browsers, such as Chrome desktop, you can change the text size in your browser settings and the size of the calculator, text and buttons will get larger or smaller proportionately.

Keyboard Control: You can use the calculator without a mouse by tabbing among the calculator keys. Press "Enter" when a key is focused. This method can be arduous however, since you must tab through all keys in sequence.

Number Keypad Control: You can use the calculator with with most Number Pads and Keyboards in the most popular browsers for numbers, clear, and the basic functions of addition, subtraction, multiplication, and division and delete/backspace.

This calculator uses a number of different equations to determine the minimum number of subjects that need to be enrolled in a study in order to have sufficient statistical power to detect a treatment effect.1

Statistical significance specifies, if a result may not be the cause of random variations within the data. But not every significant result refers to an effect with a high impact, resp. it may even describe a phenomenon that is not really perceivable in everyday life. Statistical significance mainly depends on the sample size, the quality of the data and the power of the statistical procedures. If large data sets are at hand, as it is often the case f. e. in epidemiological studies or in large scale assessments, very small effects may reach statistical significance. In order to describe, if effects have a relevant magnitude, effect sizes are used to describe the strength of a phenomenon. The most popular effect size measure surely is Cohen"s d (Cohen, 1988), but there are many more.

Here you will find a number of online calculators for the computation of different effect sizes and an interpretation table at the bottom of this page. Please click on the grey bars to show the calculators:

If the two groups have the same n, then the effect size is simply calculated by subtracting the means and dividing the result by the pooled standard deviation. The resulting effect size is called dCohen and it represents the difference between the groups in terms of their common standard deviation. It is used f. e. for comparing two experimental groups. In case, you want to do a pre-post comparison in single groups, calculator 4 or 5 should be more suitable, since they take the dependency in the data into account.

If there are relevant differences in the standard deviations, Glass suggests not to use the pooled standard deviation but the standard deviation of the control group. He argues that the standard deviation of the control group should not be influenced, at least in case of non-treatment control groups. This effect size measure is called Glass" Δ ("Glass" Delta"). Please type the data of the control group in column 2 for the correct calculation of Glass" Δ.

Finally, the Common Language Effect Size (CLES; McGraw & Wong, 1992) is a non-parametric effect size, specifying the probability that one case randomly drawn from the one sample has a higher value than a randomly drawn case from the other sample. In the calculator, we take the higher group mean as the point of reference, but you can use (1 - CLES) to reverse the view.

Analogously, the effect size can be computed for groups with different sample size, by adjusting the calculation of the pooled standard deviation with weights for the sample sizes. This approach is overall identical with dCohen with a correction of a positive bias in the pooled standard deviation. In the literature, usually this computation is called Cohen"s d as well. Please have a look at the remarks bellow the table.

The Common Language Effect Size (CLES; McGraw & Wong, 1992) is a non-parametric effect size, specifying the probability that one case randomly drawn from the one sample has a higher value than a randomly drawn case from the other sample. In the calculator, we take the higher group mean as the point of reference, but you can use (1 - CLES) to reverse the view.

Additionally, you can compute the confidence interval for the effect size and chose a desired confidence coefficient (calculation according to Hedges & Olkin, 1985, p. 86).

*Unfortunately, the terminology is imprecise on this effect size measure: Originally, Hedges and Olkin referred to Cohen and called their corrected effect size d as well. On the other hand, corrected effect sizes were called g since the beginning of the 80s. The letter is stemming from the author Glass (see Ellis, 2010, S. 27), who first suggested corrected measures. Following this logic, gHedges should be called h and not g. Usually it is simply called dCohen or gHedges to indicate, it is a corrected measure.

Intervention studies usually compare the development of at least two groups (in general an experimental group and a control group). In many cases, the pretest means and standard deviations of both groups do not match and there are a number of possibilities to deal with that problem. Klauer (2001) proposes to compute g for both groups and to subtract them afterwards. This way, different sample sizes and pre-test values are automatically corrected. The calculation is therefore equal to computing the effect sizes of both groups via form 2 and afterwards to subtract both. Morris (2008) presents different effect sizes for repeated measures designs and does a simulation study. He argues to use the pooled pretest standard deviation for weighting the differences of the pre-post-means (so called dppc2 according to Carlson & Smith, 1999). That way, the intervention does not influence the standard deviation. Additionally, there are weighting to correct for the estimation of the population effect size. Usually, Klauer (2001) and Morris (2002) yield similar results.

The downside to this approach: The pre-post-tests are not treated as repeated measures but as independent data. For dependent tests, you can use calculator 4 or 5 or 13. transform eta square from repeated measures in order to account for dependences between measurement points.

*Remarks: Klauer (2001) published his suggested effect size in German language and the reference should therefore be hard to retrieve for international readers. Klauer worked in the field of cognitive trainings and was interested in the comparison of the effectivity of different training approaches. His measure is simple and straightforward: dcorr is simply the difference between Hedge"s g of two different treatment groups in pre-post research designs. When reporting meta analytic results in international journals, it might be easier to cite Morris (2008).

While steps 1 to 3 target at comparing independent groups, especially in intervention research, the results are usually based on intra-individual changes in test scores. Morris & DeShon (2002, p.109) suggest a procedure to estimate the effect size for single-group pretest-posttest designs by taking the correlation between the pre- and post-test into account:

In case, the correlation is .5, the resulting effect size equals 1. Comparison of groups with equal size (Cohen"s d and Glass Δ). Higher values lead to an increase in the effect size. Morris & DeShon (2008) suggest to use the standard deviation of the pre-test, as this value is not influenced by the intervention, thus resembling Glass Δ. It is referred to as dRepeated Measures (dRM) in the following. The second effect size dRepeated Measures, pooled (dRM, pool) is using the pooled standard deviation, controlling for the intercorrelation of both groups (see Lakens, 2013, formula 8). Finally, another pragmatic approach, often used in meta analyses, is to simply divide the mean difference between both measurements by the averaged standard deviation without controlling for the intercorrelation - an effect size termed dav by Cummings (2012).

Effect sizes can be obtained by using the tests statistics from hypothesis tests, like Student t tests, as well. In case of independent samples, the result is essentially the same as in effect size calculation #2.

Dependent testing usually yields a higher power, because the interconnection between data points of different measurements are kept. This may be relevant f. e. when testing the same persons repeatedly, or when analyzing test results from matched persons or twins. Accordingly, more information may be used when computing effect sizes. Please note, that this approach largely has the same results compared to using a t-test statistic on gain scores and using the independent sample approach (Morris & DeShon, 2002, p. 119). Additionally, there is not THE one d, but that there are different d-like measures with different meanings. Consequently a d from an dependent sample is not directly comparable to a d from an independent sample, but yields different meanings (see notes below table).

** We would like to thank Scott Stanley for pointing out the following aspect: "When selecting "dependent" in the drop down, this calculator does not actually calculate an effect size based on accounting for the dependency between the two variables being compared. It removes that dependency already calculated into a t-statistic so formed. That is, what this calculator does is take a t value you already have, along with the correlation, from a dependent t-test and removes the effect of the dependency. That is why it returns a value more like calculator 2. This calculator will produce an effect size when dependent is selected as if you treated the data as independent even though you have a t-statistic for modeling the dependency. Some experts in meta-analysis explicitly recommend using effect sizes that are not based on taking into account the correlation. This is useful for getting to that value when that is your intention but what you are starting with is a t-test and correlation based on a dependent analysis. If you would rather have the effect size taking into account the dependency (the correlation between measures), and you have the data, you should use calculator 4." (direct correspondence on 18th of August, 2019). Further discussions on this aspect is given in Jake Westfall"s blog. To sum up: The decision on which effect size to use depends on your research question and this decision cannot be resolved definitively by the data themselves.

A very easy to interpret effect size from analyses of variance (ANOVAs) is η2 that reflects the explained proportion variance of the total variance. This proportion may be 13. transformed directly into d. If η2 is not available, the F value of the ANOVA can be used as well, as long as the sample size is known. The following computation only works for ANOVAs with two distinct groups (df1 = 1; Thalheimer & Cook, 2002):

In case, the groups means are known from ANOVAs with multiple groups, it is possible to compute the effect sizes f and d (Cohen, 1988, S. 273 ff.) and to take the dispersion of the group means into account. Prior to computing the effect size, you have to determine the minimum and maximum mean and to calculate pooled standard deviation σpool of the different groups.

Measures of effect size like d or correlations can be hard to communicate, e. g. to patients. If you use r2 f. e., effects seem to be really small and when a person does not know or understand the interpretation guidelines, even effective interventions could be seen as futile. And even small effects can be very important, as Hattie (2009) underlines:The effect of a daily dose of aspirin on cardio-vascular conditions only amounts to d = 0.07. However, if you look at the consequences, 34 of 1000 die less because of cardiac infarction.

A conversion between NNT and other effect size measures liken Cohen"s d is not easily possible. Concerning the example above, the transformation is done via the point-biserial correlation rphi which is nothing but an estimation. It leads to a constant NNT independent from the sample size and this is in line with publications like Kraemer and Kupfer (2006). Alternative approaches (comp. Furukawa & Leucht, 2011) allow to convert between d and NNT with a higher precision and usually they lead to higher numbers. The Kraemer et al. (2006) approach therefore seems to probably overestimate the effect and it seems to yield accurate results essentially, when normal distribution of the raw values is given. Please have a look at the Furukawa and Leucht (2011) paper for further information:

Studies, investigating if specific incidences occur (e. g. death, healing, academic success ...) on a binary basis (yes versus no), and if two groups differ in respect to these incidences, usually Odds Ratios, Risk Ratios and Risk Differences are used to quantify the differences between the groups (Borenstein et al. 2009, chap. 5). These forms of effect size are therefore commonly used in clinical research and in epidemiological studies:The Risk Ratio is the quotient between the risks, resp. probabilities for incidences in two different groups. The risk is computed by dividing the number of incidences by the total number in each group and building the ratio between the groups.

Cohen (1988, S. 109) suggests an effect size measure with the denomination q that permits to interpret the difference between two correlations. The two correlations are transformed with Fisher"s Z and subtracted afterwards. Cohen proposes the following categories for the interpretation: .5: large effect.

Especially in meta analytic research, it is often necessary to average correlations or to perform significance tests on the difference between correlations. Please have a look at our page Testing the Significance of Correlations for on-line calculators on these subjects.

Most statistical procedures like the computation of Cohen"s d or eta;2 at least interval scale and distribution assumptions are necessary. In case of categorical or ordinal data, often non-parametric approaches are used - in the case of statistical tests for example Wilcoxon or Mann-Whitney-U. The distributions of the their test statistics are approximated by normal distributions and finally, the result is used to assess significance. Accordingly, the test statistics can be transformed in effect sizes (comp. Fritz, Morris & Richler, 2012, p. 12; Cohen, 2008). Here you can find an effect size calculator for the test statistics of the Wilcoxon signed-rank test, Mann-Whitney-U or Kruskal-Wallis-H in order to calculate η2. You alternatively can directly use the resulting z value as well:

* Note: Please do not use the sum of the ranks but instead directly type in the test statistics U, W or z from the inferential tests. As Wilcoxon relies on dependent data, you only need to fill in the total sample size. For Kruskal-Wallis please as well specify the total sample size and the number of groups. For z, please fill in the total number of observations (either the total sample size in case of independent tests or for dependent measures with single groups the number of individuals multiplied with the number of assessments; many thanks to Helen Askell-Williams for pointing us this aspect).

Studies based on regression analysis are hard to include in meta analytic research, if they only report standardized β coefficients. It is debated, if an imputation is possible and advisable in this case. On the other hand, power of the analyses is reduced if to many studies cannot be included, which itself distorts the representativeness of the results. Peterson and Brown (2005) suggest a procedure for converting standardized β weights to r, if the β weights range between -0.5 and 0.5. r can then be used directly as an effect size or converted into d or other metrices. Peterson and Brown (2005, p. 180) conclude: "However, despite the potential usefulness of the proposed imputation approach, meta-analysts are still encouraged to make every effort to obtain original correlation coefficients."

In order to compute Cohen"s d and for other purposes, it is necessary to determine the mean (pooled) standard deviation. Here, you will find a small tool that does this for you. Different sample sizes are corrected as well and you can include up to 10 groups. Please specify the number before doing the calculation. If a value for the sample size is missing, the calculator only uses sd and does not correct for sample size.

Please choose the effect size, you want to transform, in the drop-down menu. Specify the magnitude of the effect size in the text field on the right side of the drop-down menu afterwards. The transformation is done according to Cohen (1988), Rosenthal (1994, S. 239), Borenstein, Hedges, Higgins, and Rothstein (2009; transformation of d in Odds Ratios) and Dunlap (1994; transformation in CLES).

Remark: Please consider the additional explanations concerning the transform from d to Number Needed to Treat in the section BESD and NNT. When using r as the initial effect size, the calculator draws on the formula specified by Dunlap (1994) for the conversion to CLES: CLES=arcsin(r)Π+.5. In all other case d is applied in acordance with McGraw and Wong (1992):

Here, you can see the suggestions of Cohen (1988) and Hattie (2009 S. 97) for interpreting the magnitude of effect sizes. Hattie refers to real educational contexts and therefore uses a more benignant classification, compared to Cohen. We slightly adjusted the intervals, in case, the interpretation did not exactly match the categories of the original authors.

Borenstein (2009). Effect sizes for continuous data. In H. Cooper, L. V. Hedges, & J. C. Valentine (Eds.), The handbook of research synthesis and meta analysis (pp. 221-237). New York: Russell Sage Foundation.

Borenstein, M., Hedges, L. V., Higgins, J. P. T., & Rothstein, H. R. (2009). Introduction to Meta-Analysis, Chapter 7: Converting Among Effect Sizes. Chichester, West Sussex, UK: Wiley.

Dunlap, W. P. (1994). Generalizing the common language effect size indicator to bivariate normal correlations. Psychological Bulletin, 116(3), 509-511. doi: 10.1037/0033-2909.116.3.509

Elis, P. (2010). The Essential Guide to Effect Sizes: Statistical Power, Meta-Analysis, and the Interpretation of Research Results. Cambridge: Cambridge University Press.

Fritz, C. O., Morris, P. E., & Richler, J. J. (2012). Effect size estimates: Current use, calculations, and interpretation. Journal of Experimental Psychology: General, 141(1), 2-18. https://doi.org/10.1037/a0024338

Lakens D (2013) Calculating and reporting effect sizes to facilitate cumulative science: a practical primer for t-tests and ANOVAs. Frontiers in Psychology. doi: 10.3389/fpsyg.2013.00863

Morris, S. B., & DeShon, R. P. (2002). Combining effect size estimates in meta-analysis with repeated measures and independent-groups designs. Psychological Methods, 7(1), 105-125. https://doi.org/10.1037//1082-989X.7.1.105

Morris, S. B. (2008). Estimating Effect Sizes From Pretest-Posttest-Control Group Designs. Organizational Research Methods, 11(2), 364-386. http://doi.org/10.1177/1094428106291059

Rosenthal, R. (1994). Parametric measures of effect size. In H. Cooper & L. V. Hedges (Eds.), The Handbook of Research Synthesis (231-244). New York, NY: Sage.

Thalheimer, W., & Cook, S. (2002, August). How to calculate effect sizes from published research articles: A simplified methodology. Retrieved March 9, 2014 from http://work-learning.com/effect_sizes.htm.

In case you need a reference to this page in a scientific paper, please use the following citation:Lenhard, W. & Lenhard, A. (2016). Computation of effect sizes. Retrieved from: https://www.psychometrica.de/effect_size.html. Psychometrica. DOI: 10.13140/RG.2.2.17823.92329

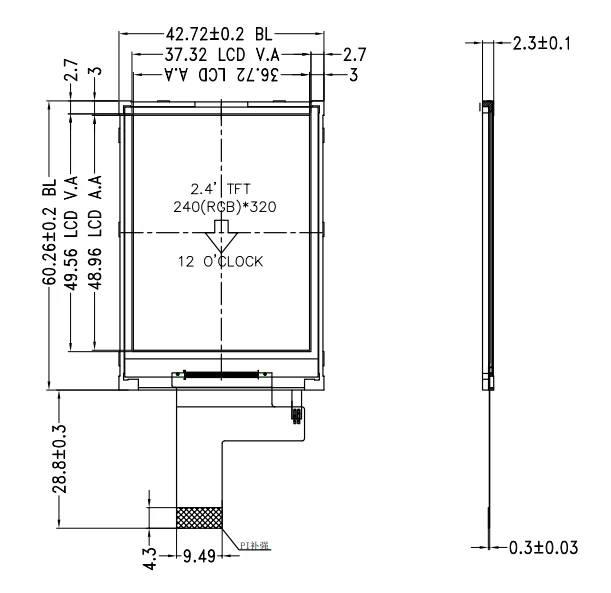
Shenzhen HZY Photoelectric Technology is a company which specialized in LCD/LCD Modules and backlight kits.As a professional manufacturer,HZY designs and produces high-quality LCD and LCM. We produce TN,HTN,BTN(VA),STN,FSTN LCD and COB,COG LCM.In addition to standard LCD,we can design and produce various kinds of custom LCD and LCM for customer as well.

Wilson’s effect-size calculator. This takes you to (Title: Dr. David B. Wilson’s online calculator)(This link open in new tab)Dr. David B. Wilson’s online calculatorthat computes a variety of effect-size indices: Cohen’s d statistic, the correlation coefficients r and V, the odds ratio, and the risk ratio. In most cases, it also provides the 95% confidence interval for the index. It is remarkably flexible, allowing users to input a wide variety of statistics to compute the desired index. For example, you can compute d by providing 30 different types of data: means and standard deviations, obtained t statistics and sample size, even mean-square errors, correlations, means, and sample size from ANCOVA. The calculator is tied to the book Practical Meta-Analysis by Mark W. Lipsey and David B. Wilson (2001) and published by Sage.

Psychometrica effect-size calculator. This takes you to an (Title: effect size calculator at the German Psychometrica site)(This link open in new tab)effect size calculator at the German Psychometrica site (don’t worry—the page is in English). Depending on the nature of your study, it allows you to compute Cohen’s d, Glass’ Δ, Hedges g, Cohen’s f, the Binomial Effect Size Display (BESD), Number Needed to Treat (NNT), odds ratio, risk ratio, and eta squared. For several scenarios it will compute confidence intervals for d. It allows users to input several different types of statistics (e.g., means, standard deviations, t values, F values, cell frequencies) to compute the desired effect size. One very useful table allows users to input a specific value of d, r, eta squared, f, or the odds ratio, and convert it into any other effect index (d, r, eta squared, f, or the odds ratio).

Psychometrica sensitivity and specificity calculator. The Psychometrica site also offers (Title: a calculator that allows users to assess the quality of predictions)(This link open in new tab)a calculator that allows users to assess the quality of predictions made on dichotomous criterion variable from a dichotomous predictor variable. Users enter frequencies into a 2 x 2 table, and the calculator reports sensitivity, specificity, hit ratio, random hit ratio, and more.

G*Power power calculator. At the following link you can (Title: download G*Power: application)(This link open in new tab)download G*Power: application for performing statistical power analysis. This is a procedure that estimates the probability that you will obtain statistically significant results given a specific sample size, the selected alpha level, and other assumptions. The software can estimate power for a wide variety of statistics, including the t test, F test, chi-square, z tests, and more. At the site you can also download a free PDF of the G*Power user manual.

AI Therapy power calculator. This takes you to (Title: AI-Therapy Statistics, a site that, among other things, offers a free online calculator)(This link open in new tab)AI-Therapy Statistics, a site that, among other things, offers a free online calculator that can compute statistic power for t tests (independent samples as well as paired samples) and one-way ANOVA (between factors as well as within-subjects). The user inputs the expected effect size, number of participant and similar information, and the calculator estimates the statistical power that the test will display.

AI Therapy sample-size calculator. The (Title: AI-Therapy Statistics site)(This link open in new tab)AI-Therapy Statistics sitealso provides a calculator that estimate the number of participants that will be needed for the results of a specific study to be statistically significant. The calculator is somewhat limited, doing this only for the independent-samples t test, paired-samples t test, and correlation coefficient. The user chooses the alpha level and inputs the expected effect size and similar information. The calculator then returns the number of participants that will be necessary to reject the null hypothesis.

This module will focus on sampling and how a sample size is determined, including statistical and non-statistical considerations. It will focus on sampling for AMR in livestock and aquatic animal health, but draw comparisons to human health where relevant, as part of the emphasis on the importance of One Health approaches in tackling AMR.