gamma correction for lcd monitors quotation

Problems like extremely poor display of shadow areas, blown-out highlights, or images prepared on Macs appearing too dark on Windows computers are often due to gamma characteristics. In this session, we"ll discuss gamma, which has a significant impact on color reproduction on LCD monitors. Understanding gamma is useful in both color management and product selection. Users who value picture quality are advised to check this information.

* Below is the translation from the Japanese of the ITmedia article "Is the Beauty of a Curve Decisive for Color Reproduction? Learning About LCD Monitor Gamma" published July 13, 2009. Copyright 2011 ITmedia Inc. All Rights Reserved.

The term gamma comes from the third letter of the Greek alphabet, written Γ in upper case and γ in lower case. The word gamma occurs often in everyday life, in terms like gamma rays, the star called Gamma Velorum, and gamma-GTP. In computer image processing, the term generally refers to the brightness of intermediate tones (gray).

Let"s discuss gamma in a little more detail. In a PC environment, the hardware used when working with color includes monitors, printers, and scanners. When using these devices connected to a PC, we input and output color information to and from each device. Since each device has its own unique color handling characteristics (or tendencies), color information cannot be output exactly as input. The color handling characteristics that arise in input and output are known as gamma characteristics.

While certain monitors are also compatible with color handling at 10 bits per RGB color (210 = 1024 tones), or 1024 x 3 (approximately 1,064,330,000 colors), operating system and application support for such monitors has lagged. Currently, some 16.77 million colors, with eight bits per RGB color, is the standard color environment for PC monitors.

When a PC and a monitor exchange color information, the ideal is a relationship in which the eight-bit color information per RGB color input from the PC to the monitor can be output accurately—that is, a 1:1 relationship for input:output. However, since gamma characteristics differ between PCs and monitors, color information is not transmitted according to a 1:1 input:output relationship.

How colors ultimately look depends on the relationship resulting from the gamma values (γ) that numerically represent the gamma characteristics of each hardware device. If the color information input is represented as x and output as y, the relationship applying the gamma value can be represented by the equation y = xγ.

Gamma characteristics are represented by the equation y = xγ. At the ideal gamma value of 1.0, y = x; but since each monitor has its own unique gamma characteristics (gamma values), y generally doesn"t equal x. The above graph depicts a curve adjusted to the standard Windows gamma value of 2.2. The standard gamma value for the Mac OS is 1.8.

Ordinarily, the nature of monitor gamma is such that intermediate tones tend to appear dark. Efforts seek to promote accurate exchange of color information by inputting data signals in which the intermediate tones have already been brightened to approach an input:output balance of 1:1. Balancing color information to match device gamma characteristics in this way is called gamma correction.

A simple gamma correction system. If we account for monitor gamma characteristics and input color information with gamma values adjusted accordingly (i.e., color information with intermediate tones brightened), color handling approaches the y = x ideal. Since gamma correction generally occurs automatically, users usually obtain correct color handling on a PC monitor without much effort. However, the precision of gamma correction varies from manufacturer to manufacturer and from product to product (see below for details).

In most cases, if a computer runs the Windows operating system, we can achieve close to ideal colors by using a monitor with a gamma value of 2.2. This is because Windows assumes a monitor with a gamma value of 2.2, the standard gamma value for Windows. Most LCD monitors are designed based on a gamma value of 2.2.

The standard monitor gamma value for the Mac OS is 1.8. The same concept applies as in Windows. We can obtain color reproduction approaching the ideal by connecting a Mac to a monitor configured with a gamma value of 1.8.

An example of the same image displayed at gamma values of 2.2 (photo at left) and 1.8 (photo at right). At a gamma value of 1.8, the overall image appears brighter. The LCD monitor used is EIZO"s 20-inch wide-screen EV2023W FlexScan model (ITmedia site).

To equalize color handling in mixed Windows and Mac environments, it"s a good idea to standardize the gamma values between the two operating systems. Changing the gamma value for the Mac OS is easy; but Windows provides no such standard feature. Since Windows users perform color adjustments through the graphics card driver or separate color-adjustment software, changing the gamma value can be an unexpectedly complex task. If the monitor used in a Windows environment offers a feature for adjusting gamma values, obtaining more accurate results will likely be easier.

If we know that a certain image was created in a Mac OS environment with a gamma value of 1.8, or if an image received from a Mac user appears unnaturally dark, changing the monitor gamma setting to 1.8 should show the image with the colors intended by the creator.

Eizo Nanao"s LCD monitors allow users to configure the gamma value from the OSD menu, making this procedure easy. In addition to the initially configured gamma value of 2.2., one can choose from multiple settings, including the Mac OS standard of 1.8.

To digress slightly, standard gamma values differ between Windows and Mac OS for reasons related to the design concepts and histories of the two operating systems. Windows adopted a gamma value corresponding to television (2.2), while the Mac OS adopted a gamma value corresponding to commercial printers (1.8). The Mac OS has a long history of association with commercial printing and desktop publishing applications, for which 1.8 remains the basic gamma value, even now. On the other hand, a gamma value of 2.2 is standard in the sRGB color space, the standard for the Internet and for digital content generally, and for Adobe RGB, the use of which has expanded for wide-gamut printing,.

Given the proliferating use of color spaces like sRGB and Adobe RGB, plans call for the latest Mac OS scheduled for release by Apple Computer in September 2009, Mac OS X 10.6 Snow Leopard, to switch from a default gamma value of 1.8 to 2.2. A gamma value of 2.2 is expected to become the future mainstream for Macs.

On the preceding page, we mentioned that the standard gamma value in a Windows environment is 2.2 and that many LCD monitors can be adjusted to a gamma value of 2.2. However, due to the individual tendencies of LCD monitors (or the LCD panels installed in them), it"s hard to graph a smooth gamma curve of 2.2.

Traditionally, LCD panels have featured S-shaped gamma curves, with ups and downs here and there and curves that diverge by RGB color. This phenomenon is particularly marked for dark and light tones, often appearing to the eye of the user as tone jumps, color deviations, and color breakdown.

The internal gamma correction feature incorporated into LCD monitors that emphasize picture quality allows such irregularity in the gamma curve to be corrected to approach the ideal of y = x γ. Device specs provide one especially useful figure to help us determine whether a monitor has an internal gamma correction feature: A monitor can be considered compatible with internal gamma correction if the figure for maximum number of colors is approximately 1,064,330,000 or 68 billion or if the specs indicate the look-up table (LUT) is 10- or 12-bit.

An internal gamma correction feature applies multi-gradation to colors and reallocates them. While the input from a PC to an LCD monitor is in the form of color information at eight bits per RGB color, within the LCD monitor, multi-gradation is applied to increase this to 10 bits (approximately 1,064,330,000 colors) or 12 bits (approximately 68 billion colors). The optimal color at eight bits per RGB color (approximately 16.77 million colors) is identified by referring to the LUT and displayed on screen. This corrects irregularity in the gamma curve and deviations in each RGB color, causing the output on screen to approach the ideal of y = x γ.

Let"s look at a little more information on the LUT. The LUT is a table containing the results of certain calculations performed in advance. The results for certain calculations can be obtained simply by referring to the LUT, without actually performing the calculations. This accelerates processing and reduces the load on a system. The LUT in an LCD monitor identifies the optimal eight-bit RGB colors from multi-gradation color data of 10 or more bits.

An overview of an internal gamma correction feature. Eight-bit RGB color information input from the PC is subjected to multi-gradation to 10 or more bits. This is then remapped to the optimal eight-bit RGB tone by referring to the LUT. Following internal gamma correction, the results approach the ideal gamma curve, dramatically improving on screen gradation and color reproduction.

Eizo Nanao"s LCD monitors proactively employ internal gamma correction features. In models designed especially for high picture quality and in some models in the ColorEdge series designed for color management, eight-bit RGB input signals from the PC are subjected to multi-gradation, and calculations are performed at 14 or 16 bits. A key reason for performing calculations at bit counts higher than the LUT bit count is to improve gradation still further, particularly the reproduction of darker tones. Users seeking high-quality color reproduction should probably choose a monitor model like this one.

In conclusion, we"ve prepared image patterns that make it easy to check the gamma values of an LCD monitor, based on this session"s discussion. Looking directly at your LCD monitor, move back slightly from the screen and gaze at the following images with your eyes half-closed. Visually compare the square outlines and the stripes around them, looking for patterns that appear to have the same tone of gray (brightness). The pattern for which the square frame and the striped pattern around it appear closest in brightness represents the rough gamma value to which the monitor is currently configured.

Based on a gamma value of 2.2, if the square frame appears dark, the LCD monitor"s gamma value is low. If the square frame appears bright, the gamma value is high. You can adjust the gamma value by changing the LCD monitor"s brightness settings or by adjusting brightness in the driver menu for the graphics card.

Naturally, it"s even easier to adjust the gamma if you use a model designed for gamma value adjustments, like an EIZO LCD monitor. For even better color reproduction, you can set the gamma value and optimize color reproduction by calibrating your monitor.

Many statements on here are incorrect. Gamma in the signal path is a desired benefit, and a design choice by early video engineers to reduce perceived noise in transmission.

All vacuum tubes, CRTs included, exhibit various non-linearities (see Langmuir-Child law) CRTs can vary from a "gamma" of 1.5 to over 3.5 (when driven by a voltage signal) depending on various design differences. The nonlinearities were less of an issue with monochrome, but became more critical with color so the NTSC specified a signal gamma of 1/2.2. CRT design and supporting circuits adjust actual gamma from the Langmuir-Child law (commonly understood as 1.5, but is typically higher with CRTs due to a number of factors) to a level in line with human perception "gamma" of ~2.5. For NTSC, the television set was assumed to have a gamma target of ~2.4,** while PAL indicated ~2.8

The higher gamma in the old analog broadcast signal standards is specifically to reduce perceived noise, based on human perception being non-linear. In this use case, taking advantage of the non-linearities to hide noise by the "companding" effect of gamma encoding the signal. This is quite academic.

There are a few ways that CRT TV & Monitor design could have been altered to achieve linearity as opposed to a gamma-type curve, but a gamma curve in analog broadcasting reduced apparent noise by 30 dB. Gamma was desirable then AS IT IS NOW.

Gamma is needed even if an LCD monitor could be used in a linear (gamma 1.0) way. The claims here that gamma is no longer needed are complete bunk, and fail to understand the current purpose of applying a pre-emphasis curve.

The computer monitors we use are still either 8 or 10 bit FOR DISPLAY, so all linear images still need to be gamma-adjusted before being sent to the monitor. Why?

Most "good" monitors are just 8 bit per chan, and many are just "6 bit internal" meaning they take an 8 bit per chan image and display as 6 bit per channel. How can they make an acceptable image?

10 bit per channel monitors are rare and expensive (like my NEX PA271W). My NEC can take a 10 bit signal, and uses a 14 bit internal LUT for profiling. But 10 bits is still not enough for linear!

Gamma or some form of preemph/deemph curve is required even for 10 bit. 12 bit is the bare minimum for reasonable linear display, and even then is unacceptable for the feature film industry.

DCI was created for theaters and is its own closed eco-system, with no reliance on old technologies like CRT. If there was some "advantage" to using a linear (gamma 1.0) space, it would have been used, but it is not.

** While the NTSC specified a signal gamma of 1/2.2, TVs were expected to have a gamma of 2.4 for a system gamma gain. It is useful to point out that Rec709 (HDTV) and sRGB are identical except for the transfer curve. And interestingly, Rec709 (via BT1886) specifies a "physical display gamma" of 2.4 (i.e. the gamma of the monitor itself) and sRGB monitors are typically set at 2.4 or higher (surveys show most users set them 2.5 and up). But the SIGNAL gamma is different, approx. 1/2.2 for sRGB and approx 1/2.0 for Rec709. in both cases, there is a system gamma gain which is intentional based on the expected viewing environment.

The effect of gamma correction on an image: The original image was taken to varying powers, showing that powers larger than 1 make the shadows darker, while powers smaller than 1 make dark regions lighter.

Gamma correction or gamma is a nonlinear operation used to encode and decode luminance or tristimulus values in video or still image systems.power-law expression:

Gamma encoding of images is used to optimize the usage of bits when encoding an image, or bandwidth used to transport an image, by taking advantage of the non-linear manner in which humans perceive light and color.lightness), under common illumination conditions (neither pitch black nor blindingly bright), follows an approximate power function (which has no relation to the gamma function), with greater sensitivity to relative differences between darker tones than between lighter tones, consistent with the Stevens power law for brightness perception. If images are not gamma-encoded, they allocate too many bits or too much bandwidth to highlights that humans cannot differentiate, and too few bits or too little bandwidth to shadow values that humans are sensitive to and would require more bits/bandwidth to maintain the same visual quality.floating-point images is not required (and may be counterproductive), because the floating-point format already provides a piecewise linear approximation of a logarithmic curve.

Although gamma encoding was developed originally to compensate for the input–output characteristic of cathode ray tube (CRT) displays, it is not its main purpose or advantage in modern systems. In CRT displays, the light intensity varies nonlinearly with the electron-gun voltage. Altering the input signal by gamma compression can cancel this nonlinearity, such that the output picture has the intended luminance. However, the gamma characteristics of the display device do not play a factor in the gamma encoding of images and video. They need gamma encoding to maximize the visual quality of the signal, regardless of the gamma characteristics of the display device.

Analogously, digital cameras record light using electronic sensors that usually respond linearly. In the process of rendering linear raw data to conventional RGB data (e.g. for storage into JPEG image format), color space transformations and rendering transformations will be performed. In particular, almost all standard RGB color spaces and file formats use a non-linear encoding (a gamma compression) of the intended intensities of the primary colors of the photographic reproduction. In addition, the intended reproduction is almost always nonlinearly related to the measured scene intensities, via a tone reproduction nonlinearity.

That is, gamma can be visualized as the slope of the input–output curve when plotted on logarithmic axes. For a power-law curve, this slope is constant, but the idea can be extended to any type of curve, in which case gamma (strictly speaking, "point gamma"

When a photographic film is exposed to light, the result of the exposure can be represented on a graph showing log of exposure on the horizontal axis, and density, or negative log of transmittance, on the vertical axis. For a given film formulation and processing method, this curve is its characteristic or Hurter–Driffield curve.

Output to CRT-based television receivers and monitors does not usually require further gamma correction. The standard video signals that are transmitted or stored in image files incorporate gamma compression matching the gamma expansion of the CRT (although it is not the exact inverse).

For television signals, gamma values are fixed and defined by the analog video standards. CCIR System M and N, associated with NTSC color, use gamma 2.2; the rest (systems B/G, H, I, D/K, K1 and L) associated with PAL or SECAM color, use gamma 2.8.

In most computer display systems, images are encoded with a gamma of about 0.45 and decoded with the reciprocal gamma of 2.2. A notable exception, until the release of Mac OS X 10.6 (Snow Leopard) in September 2009, were Macintosh computers, which encoded with a gamma of 0.55 and decoded with a gamma of 1.8. In any case, binary data in still image files (such as JPEG) are explicitly encoded (that is, they carry gamma-encoded values, not linear intensities), as are motion picture files (such as MPEG). The system can optionally further manage both cases, through color management, if a better match to the output device gamma is required.

Plot of the sRGB standard gamma-expansion nonlinearity in red, and its local gamma value (slope in log–log space) in blue. The local gamma rises from 1 to about 2.2.

The sRGB color space standard used with most cameras, PCs, and printers does not use a simple power-law nonlinearity as above, but has a decoding gamma value near 2.2 over much of its range, as shown in the plot to the right. Below a compressed value of 0.04045 or a linear intensity of 0.00313, the curve is linear (encoded value proportional to intensity), so γ = 1. The dashed black curve behind the red curve is a standard γ = 2.2 power-law curve, for comparison.

Gamma correction in computers is used, for example, to display a gamma = 1.8 Apple picture correctly on a gamma = 2.2 PC monitor by changing the image gamma. Another usage is equalizing of the individual color-channel gammas to correct for monitor discrepancies.

Some picture formats allow an image"s intended gamma (of transformations between encoded image samples and light output) to be stored as metadata, facilitating automatic gamma correction as long as the display system"s exponent is known. The PNG specification includes the gAMA chunk for this purposeJPEG and TIFF the Exif Gamma tag can be used.

These features have historically caused problems, especially on the web. There is no numerical value of gamma that matches the "show the 8-bit numbers unchanged" method used for JPG, GIF, HTML, and CSS colors, so the PNG would not match.Google Chrome (and all other Chromium-based browsers) and Mozilla Firefox either ignore the gamma setting entirely, or ignore it when set to known wrong values.

A gamma characteristic is a power-law relationship that approximates the relationship between the encoded luma in a television system and the actual desired image luminance.

With this nonlinear relationship, equal steps in encoded luminance correspond roughly to subjectively equal steps in brightness. Ebner and Fairchildused an exponent of 0.43 to convert linear intensity into lightness (luma) for neutrals; the reciprocal, approximately 2.33 (quite close to the 2.2 figure cited for a typical display subsystem), was found to provide approximately optimal perceptual encoding of grays.

The following illustration shows the difference between a scale with linearly-increasing encoded luminance signal (linear gamma-compressed luma input) and a scale with linearly-increasing intensity scale (linear luminance output).

On most displays (those with gamma of about 2.2), one can observe that the linear-intensity scale has a large jump in perceived brightness between the intensity values 0.0 and 0.1, while the steps at the higher end of the scale are hardly perceptible. The gamma-encoded scale, which has a nonlinearly-increasing intensity, will show much more even steps in perceived brightness.

A cathode ray tube (CRT), for example, converts a video signal to light in a nonlinear way, because the electron gun"s intensity (brightness) as a function of applied video voltage is nonlinear. The light intensity I is related to the source voltage Vs according to

where γ is the Greek letter gamma. For a CRT, the gamma that relates brightness to voltage is usually in the range 2.35 to 2.55; video look-up tables in computers usually adjust the system gamma to the range 1.8 to 2.2,

For simplicity, consider the example of a monochrome CRT. In this case, when a video signal of 0.5 (representing a mid-gray) is fed to the display, the intensity or brightness is about 0.22 (resulting in a mid-gray, about 22% the intensity of white). Pure black (0.0) and pure white (1.0) are the only shades that are unaffected by gamma.

To compensate for this effect, the inverse transfer function (gamma correction) is sometimes applied to the video signal so that the end-to-end response is linear. In other words, the transmitted signal is deliberately distorted so that, after it has been distorted again by the display device, the viewer sees the correct brightness. The inverse of the function above is

where Vc is the corrected voltage, and Vs is the source voltage, for example, from an image sensor that converts photocharge linearly to a voltage. In our CRT example 1/γ is 1/2.2 ≈ 0.45.

A color CRT receives three video signals (red, green, and blue) and in general each color has its own value of gamma, denoted γR, γG or γB. However, in simple display systems, a single value of γ is used for all three colors.

Other display devices have different values of gamma: for example, a Game Boy Advance display has a gamma between 3 and 4 depending on lighting conditions. In LCDs such as those on laptop computers, the relation between the signal voltage Vs and the intensity I is very nonlinear and cannot be described with gamma value. However, such displays apply a correction onto the signal voltage in order to approximately get a standard γ = 2.5 behavior. In NTSC television recording, γ = 2.2.

The power-law function, or its inverse, has a slope of infinity at zero. This leads to problems in converting from and to a gamma colorspace. For this reason most formally defined colorspaces such as sRGB will define a straight-line segment near zero and add raising x + K (where K is a constant) to a power so the curve has continuous slope. This straight line does not represent what the CRT does, but does make the rest of the curve more closely match the effect of ambient light on the CRT. In such expressions the exponent is not the gamma; for instance, the sRGB function uses a power of 2.4 in it, but more closely resembles a power-law function with an exponent of 2.2, without a linear portion.

Up to four elements can be manipulated in order to achieve gamma encoding to correct the image to be shown on a typical 2.2- or 1.8-gamma computer display:

The pixel"s intensity values in a given image file; that is, the binary pixel values are stored in the file in such way that they represent the light intensity via gamma-compressed values instead of a linear encoding. This is done systematically with digital video files (as those in a DVD movie), in order to minimize the gamma-decoding step while playing, and maximize image quality for the given storage. Similarly, pixel values in standard image file formats are usually gamma-compensated, either for sRGB gamma (or equivalent, an approximation of typical of legacy monitor gammas), or according to some gamma specified by metadata such as an ICC profile. If the encoding gamma does not match the reproduction system"s gamma, further correction may be done, either on display or to create a modified image file with a different profile.

The rendering software writes gamma-encoded pixel binary values directly to the video memory (when highcolor/truecolor modes are used) or in the CLUT hardware registers (when indexed color modes are used) of the display adapter. They drive Digital-to-Analog Converters (DAC) which output the proportional voltages to the display. For example, when using 24-bit RGB color (8 bits per channel), writing a value of 128 (rounded midpoint of the 0–255 byte range) in video memory it outputs the proportional ≈ 0.5 voltage to the display, which it is shown darker due to the monitor behavior. Alternatively, to achieve ≈ 50% intensity, a gamma-encoded look-up table can be applied to write a value near to 187 instead of 128 by the rendering software.

Modern display adapters have dedicated calibrating CLUTs, which can be loaded once with the appropriate gamma-correction look-up table in order to modify the encoded signals digitally before the DACs that output voltages to the monitor.hardware calibration.

Some modern monitors allow the user to manipulate their gamma behavior (as if it were merely another brightness/contrast-like setting), encoding the input signals by themselves before they are displayed on screen. This is also a calibration by hardware technique but it is performed on the analog electric signals instead of remapping the digital values, as in the previous cases.

In a typical system, for example from camera through JPEG file to display, the role of gamma correction will involve several cooperating parts. The camera encodes its rendered image into the JPEG file using one of the standard gamma values such as 2.2, for storage and transmission. The display computer may use a color management engine to convert to a different color space (such as older Macintosh"s γ = 1.8 color space) before putting pixel values into its video memory. The monitor may do its own gamma correction to match the CRT gamma to that used by the video system. Coordinating the components via standard interfaces with default standard gamma values makes it possible to get such system properly configured.

This procedure is useful for making a monitor display images approximately correctly, on systems in which profiles are not used (for example, the Firefox browser prior to version 3.0 and many others) or in systems that assume untagged source images are in the sRGB colorspace.

In the test pattern, the intensity of each solid color bar is intended to be the average of the intensities in the surrounding striped dither; therefore, ideally, the solid areas and the dithers should appear equally bright in a system properly adjusted to the indicated gamma.

Normally a graphics card has contrast and brightness control and a transmissive LCD monitor has contrast, brightness, and backlight control. Graphics card and monitor contrast and brightness have an influence on effective gamma, and should not be changed after gamma correction is completed.

Given a desired display-system gamma, if the observer sees the same brightness in the checkered part and in the homogeneous part of every colored area, then the gamma correction is approximately correct.

Before gamma correction the desired gamma and color temperature should be set using the monitor controls. Using the controls for gamma, contrast and brightness, the gamma correction on an LCD can only be done for one specific vertical viewing angle, which implies one specific horizontal line on the monitor, at one specific brightness and contrast level. An ICC profile allows one to adjust the monitor for several brightness levels. The quality (and price) of the monitor determines how much deviation of this operating point still gives a satisfactory gamma correction. Twisted nematic (TN) displays with 6-bit color depth per primary color have lowest quality. In-plane switching (IPS) displays with typically 8-bit color depth are better. Good monitors have 10-bit color depth, have hardware color management and allow hardware calibration with a tristimulus colorimeter. Often a 6bit plus FRC panel is sold as 8bit and a 8bit plus FRC panel is sold as 10bit. FRC is no true replacement for more bits. The 24-bit and 32-bit color depth formats have 8 bits per primary color.

With Microsoft Windows 7 and above the user can set the gamma correction through the display color calibration tool dccw.exe or other programs.ICC profile file and load it as default. This makes color management easy.color Look Up Table correctly after waking up from standby or hibernate mode and show wrong gamma. In this case update the graphics card driver.

On some operating systems running the X Window System, one can set the gamma correction factor (applied to the existing gamma value) by issuing the command xgamma -gamma 0.9 for setting gamma correction factor to 0.9, and xgamma for querying current value of that factor (the default is 1.0). In macOS systems, the gamma and other related screen calibrations are made through the System Preferences.

The test image is only valid when displayed "raw", i.e. without scaling (1:1 pixel to screen) and color adjustment, on the screen. It does, however, also serve to point out another widespread problem in software: many programs perform scaling in a color space with gamma, instead of a physically-correct linear space. In a sRGB color space with an approximate gamma of 2.2, the image should show a "2.2" result at 50% size, if the zooming is done linearly. Jonas Berlin has created a "your scaling software sucks/rules" image based on the same principle.

In addition to scaling, the problem also applies to other forms of downsampling (scaling down), such as chroma subsampling in JPEG"s gamma-enabled Y′CbCr.WebP solves this problem by calculating the chroma averages in linear space then converting back to a gamma-enabled space; an iterative solution is used for larger images. The same "sharp YUV" (formerly "smart YUV") code is used in sjpeg. Kornelski provides a simpler approximation by luma-based weighted average.Alpha compositing, color gradients, and 3D rendering are also affected by this issue.

Paradoxically, when upsampling (scaling up) an image, the result processed in the "wrong" gamma-enabled space tends to be more aesthetically pleasing. This is because upscaling filters are tuned to minimize the ringing artifacts in a linear space, but human perception is non-linear and better approximated by gamma. An alternative way to trim the artifacts is using a sigmoidal light transfer function, a technique pioneered by GIMP"s LoHalo filter and later adopted by madVR.

The term intensity refers strictly to the amount of light that is emitted per unit of time and per unit of surface, in units of lux. Note, however, that in many fields of science this quantity is called luminous exitance, as opposed to luminous intensity, which is a different quantity. These distinctions, however, are largely irrelevant to gamma compression, which is applicable to any sort of normalized linear intensity-like scale.

One contrasts relative luminance in the sense of color (no gamma compression) with luma in the sense of video (with gamma compression), and denote relative luminance by Y and luma by Y′, the prime symbol (′) denoting gamma compression.

Gamma correction is a type of power law function whose exponent is the Greek letter gamma (γ). It should not be confused with the mathematical Gamma function. The lower case gamma, γ, is a parameter of the former; the upper case letter, Γ, is the name of (and symbol used for) the latter (as in Γ(x)). To use the word "function" in conjunction with gamma correction, one may avoid confusion by saying "generalized power law function".

Without context, a value labeled gamma might be either the encoding or the decoding value. Caution must be taken to correctly interpret the value as that to be applied-to-compensate or to be compensated-by-applying its inverse. In common parlance, in many occasions the decoding value (as 2.2) is employed as if it were the encoding value, instead of its inverse (1/2.2 in this case), which is the real value that must be applied to encode gamma.

McKesson, Jason L. "Chapter 12. Dynamic Range – Linearity and Gamma". Learning Modern 3D Graphics Programming. Archived from the original on 18 July 2013. Retrieved 11 July 2013.

"11A: Characteristics of systems for monochrome and color television". Reports of the CCIR, 1990: Also Decisions : XVIIth Plenary Assembly, Dusseldorf (PDF). International Radio Consultative Committee. 1990.

Fritz Ebner and Mark D Fairchild, "Development and testing of a color space (IPT) with improved hue uniformity," Proceedings of IS&T/SID"s Sixth Color Imaging Conference, p 8-13 (1998).

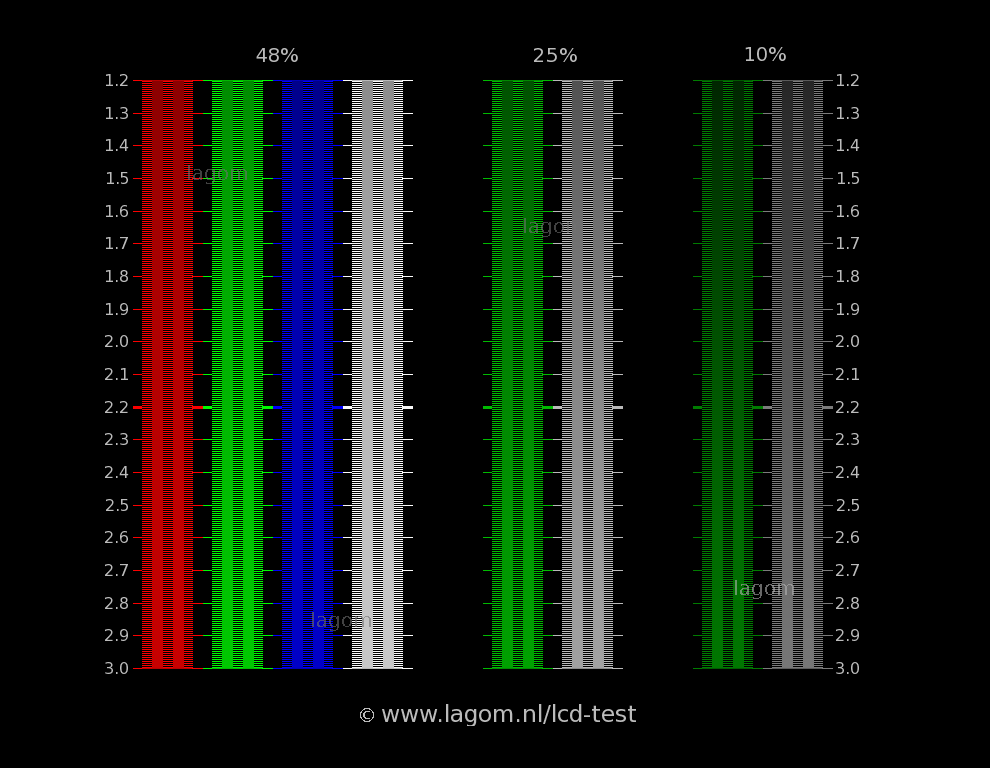
Koren, Norman. "Monitor calibration and gamma". Retrieved 2018-12-10. The chart below enables you to set the black level (brightness) and estimate display gamma over a range of 1 to 3 with precison better than 0.1.

Nienhuys, Han-Kwang (2008). "Gamma calibration". Retrieved 2018-11-30. The reason for using 48% rather than 50% as a luminance is that many LCD screens have saturation issues in the last 5 percent of their brightness range that would distort the gamma measurement.

Andrews, Peter. "The Monitor calibration and Gamma assessment page". Retrieved 2018-11-30. the problem is caused by the risetime of most monitor hardware not being sufficiently fast to turn from full black to full white in the space of a single pixel, or even two, in some cases.

Werle, Eberhard. "Quickgamma". Retrieved 2018-12-10. QuickGamma is a small utility program to calibrate a monitor on the fly without having to buy expensive hardware tools.

With most display systems, the gamma correction is applied in the video card (by downloading a custom LUT into the card). Some of the higher-end monitors (high end NEC & Eizo monitors) have the ability to apply a correction LUT internally inside the monitor. The advantage of this is that they are generally higher bit depth (typically 10 or 12 bits) than the correction applied via the display card (8-bits).

As it seems from FreddyNZ"s calibration image my LCD monitor is totally "screwed up".As freddyNZ mentioned, the correction LUT isn"t automatically calculated, as it depends upon the individual characteristics of the display (CRT or LCD) being used. If you"re using a hardware display calibrator, it starts off by loading a linear LUT into the video card and then proceeds to measure the characteristics of the display. It then calulates a correction LUT that will bring the display to a known gamma & color temperature and loads that into the video card (or monitor if supported). After calibrating the display to known set of values, it then proceeds to characterize the color characteristics of the display. This data is used to build the ICC profile for the display. Note that if you use a display that doesn"t support an internal correction LUT (that"s most of us), the correction LUT gets loaded into the video card when the OS boots up. It is usually done with a small LUT loader utility which runs at startup and reads the correction LUT from the default display profile and loads it into the video card.

Do you happen to know if a correction LUT made by a hardware calibrator is stored inside the ICC profile you mention? Or are the monitor"s ICC profile and the correction LUT two totally different things?

"When the data is saved, after being gamma corrected to 1.8, that gamma correction stays with the file. However, most file formats (GIF, JPEG) don"t have anyway to tell a user the gamma correction that has already been applied to image data. Therefore, the user must guess and gamma correct until he is satisfied with how it looks. The Targa and PNG file formats do encode the exact gamma information, removing some of the guess work. The 3D modeling program, 3D Studio, actually takes advantage of this information!

Gamma correction, then, can be done on file data directly (the individual bits in the file are changed to reflect the correction). This is what is meant by the File Gamma or "gamma of a file." On the other hand gamma correction can be done as post processing on file data. In the latter case, the data in the file is unchanged, but between reading the file and displaying the data on your monitor, the data is gamma corrected for display purposes. Ideally, if one knows the File Gamma and their own System Gamma, they can determine the gamma correction needed (if any) to accurately display the file on their system."

So are gamma corrected values stored often in image files by image editing software? And do any programs really take it into consideration when showing this kind of image file by lowering midtones of the image before sending it to video card? So is this "file gamma" really a common problem among photo editing or a very rare exception?

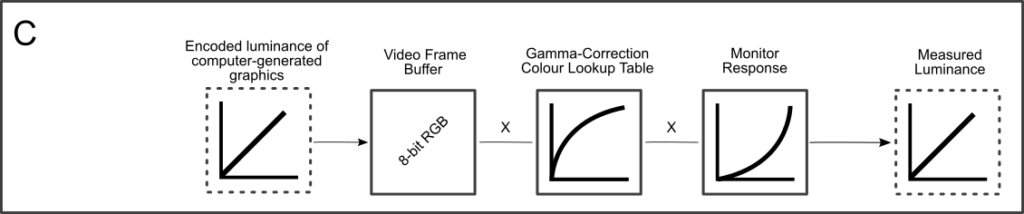
Basically, gamma is the relationship between the brightness of a pixel as it appears on the screen, and the numerical value of that pixel. Generally Gamma is just about defining relationships.

Different display devices (monitor, phone screen, TV) do not display luminance correctly neither. So, one needs to correct them, therefore the gamma correction function.

The human perception of brightness, under common illumination conditions (not pitch black nor blindingly bright), follows an approximate power function (note: no relation to the gamma function), with greater sensitivity to relative differences between darker tones than between lighter ones, consistent with the Stevens’ power law for brightness perception. If images are not gamma-encoded, they allocate too many bits or too much bandwidth to highlights that humans cannot differentiate, and too few bits or too little bandwidth to shadow values that humans are sensitive to and would require more bits/bandwidth to maintain the same visual quality.

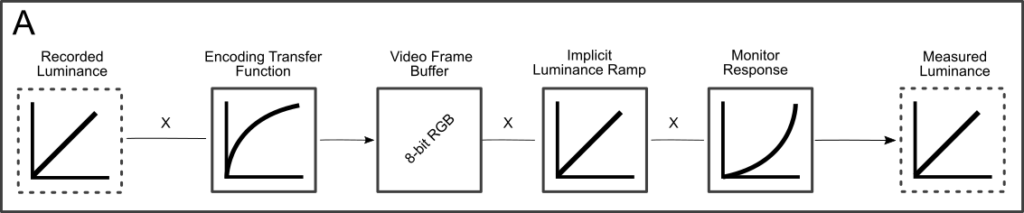
A gamma encoded image has to have “gamma correction” applied when it is viewed — which effectively converts it back into light from the original scene. In other words, the purpose of gamma encoding is for recording the image — not for displaying the image. Fortunately this second step (the “display gamma”) is automatically performed by your monitor and video card. The following diagram illustrates how all of this fits together:

The display gamma can be a little confusing because this term is often used interchangeably with gamma correction, since it corrects for the file gamma. This is the gamma that you are controlling when you perform monitor calibration and adjust your contrast setting. Fortunately, the industry has converged on a standard display gamma of 2.2, so one doesn’t need to worry about the pros/cons of different values.

Gamma encoding of images is used to optimize the usage of bits when encoding an image, or bandwidth used to transport an image, by taking advantage of the non-linear manner in which humans perceive light and color. Human response to luminance is also biased. Especially sensible to dark areas.

You probably already know that a pixel can have any ‘value’ of Red, Green, and Blue between 0 and 255, and you would therefore think that a pixel value of 127 would appear as half of the maximum possible brightness, and that a value of 64 would represent one-quarter brightness, and so on. Well, that’s just not the case.

Because the resulting linear image in not suitable for viewing, but contains all the proper data. Pixar’s IT viewer can compensate by showing the rendered image through a sRGB look up table (LUT), which is identical to what will be the final image after the sRGB gamma curve is applied in post.

This would be simple enough if every software would play by the same rules, but they don’t. In fact, the default gamma workflow for many 3D software is incorrect. This is where the knowledge of a proper imaging workflow comes in to save the day.

Cathode-ray tubes have a peculiar relationship between the voltage applied to them, and the amount of light emitted. It isn’t linear, and in fact it follows what’s called by mathematicians and other geeks, a ‘power law’ (a number raised to a power). The numerical value of that power is what we call the gamma of the monitor or system.

Thus. Gamma describes the nonlinear relationship between the pixel levels in your computer and the luminance of your monitor (the light energy it emits) or the reflectance of your prints. The equation is,

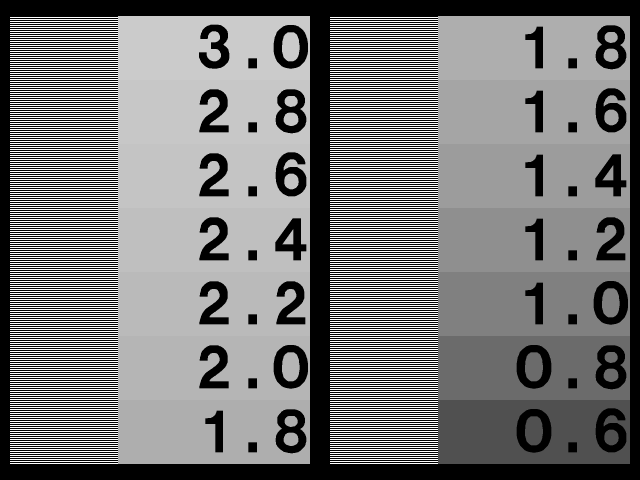
– Black level is set by the (misnamed) monitor Brightness control. The relationship is linear if gamma = 1. The chart illustrates the relationship for gamma = 1, 1.5, 1.8 and 2.2 with C = 1 and black level = 0.

Gamma affects middle tones; it has no effect on black or white. If gamma is set too high, middle tones appear too dark. Conversely, if it’s set too low, middle tones appear too light.

The native gamma of monitors – the relationship between grid voltage and luminance – is typically around 2.5, though it can vary considerably. This is well above any of the display standards, so you must be aware of gamma and correct it.

Video cameras have gammas of approximately 0.45 – the inverse of 2.2. The viewing or system gamma is the product of the gammas of all the devices in the system – the image acquisition device (film+scanner or digital camera), color lookup table (LUT), and monitor. System gamma is typically between 1.1 and 1.5. Viewing flare and other factor make images look flat at system gamma = 1.0.

CRT Monitors. Due to an odd bit of engineering luck, the native gamma of a CRT is 2.5 — almost the inverse of our eyes. Values from a gamma-encoded file could therefore be sent straight to the screen and they would automatically be corrected and appear nearly OK. However, a small gamma correction of ~1/1.1 needs to be applied to achieve an overall display gamma of 2.2. This is usually already set by the manufacturer’s default settings, but can also be set during monitor calibration.

LCD Monitors. LCD monitors weren’t so fortunate; ensuring an overall display gamma of 2.2 often requires substantial corrections, and they are also much less consistent than CRT’s. LCDs therefore require something called a look-up table (LUT) in order to ensure that input values are depicted using the intended display gamma (amongst other things). See the tutorial on monitor calibration: look-up tables for more on this topic.

About black level (brightness). Your monitor’s brightness control (which should actually be called black level) can be adjusted using the mostly black pattern on the right side of the chart. This pattern contains two dark gray vertical bars, A and B, which increase in luminance with increasing gamma. (If you can’t see them, your black level is way low.) The left bar (A) should be just above the threshold of visibility opposite your chosen gamma (2.2 or 1.8) – it should be invisible where gamma is lower by about 0.3. The right bar (B) should be distinctly visible: brighter than (A), but still very dark. This chart is only for monitors; it doesn’t work on printed media.

The 1.8 and 2.2 gray patterns at the bottom of the image represent a test of monitor quality and calibration. If your monitor is functioning properly and calibrated to gamma = 2.2 or 1.8, the corresponding pattern will appear smooth neutral gray when viewed from a distance. Any waviness, irregularity, or color banding indicates incorrect monitor calibration or poor performance.

Another test to see whether one’s computer monitor is properly hardware adjusted and can display shadow detail in sRGB images properly, they should see the left half of the circle in the large black square very faintly but the right half should be clearly visible. If not, one can adjust their monitor’s contrast and/or brightness setting. This alters the monitor’s perceived gamma. The image is best viewed against a black background.

This procedure is not suitable for calibrating or print-proofing a monitor. It can be useful for making a monitor display sRGB images approximately correctly, on systems in which profiles are not used (for example, the Firefox browser prior to version 3.0 and many others) or in systems that assume untagged source images are in the sRGB colorspace.

On some operating systems running the X Window System, one can set the gamma correction factor (applied to the existing gamma value) by issuing the command xgamma -gamma 0.9 for setting gamma correction factor to 0.9, and xgamma for querying current value of that factor (the default is 1.0). In OS X systems, the gamma and other related screen calibrations are made through the System Preference

Linear color space means that numerical intensity values correspond proportionally to their perceived intensity. This means that the colors can be added and multiplied correctly. A color space without that property is called ”non-linear”. Below is an example where an intensity value is doubled in a linear and a non-linear color space. While the corresponding numerical values in linear space are correct, in the non-linear space (gamma = 0.45, more on this later) we can’t simply double the value to get the correct intensity.

The need for gamma arises for two main reasons: The first is that screens have been built with a non-linear response to intensity. The other is that the human eye can tell the difference between darker shades better than lighter shades. This means that when images are compressed to save space, we want to have greater accuracy for dark intensities at the expense of lighter intensities. Both of these problems are resolved using gamma correction, which is to say the intensity of every pixel in an image is put through a power function. Specifically, gamma is the name given to the power applied to the image.

CRT screens, simply by how they work, apply a gamma of around 2.2, and modern LCD screens are designed to mimic that behavior. A gamma of 2.2, the reciprocal of 0.45, when applied to the brightened images will darken them, leaving the original image.

If your textures are stored in the more common image file formats, they will contain the values as they are presented to the graphics scanout. Now there are two common hardware scenarios:

The scanout interface outputs a linear signal and the display device will then internally apply a nonlinear mapping. Old CRT monitors were nonlinear due to their physics: The amplifiers could put only so much current into the electron beam, the phosphor saturating and so on – that"s why the whole gamma thing was introduced in the first place, to model the nonlinearities of CRT displays.

Some devices however are linear, and ask the image producing device to supply a proper matching LUT for the desired output color profile on the scanout.

For illustration I quickly hooked up my laptop"s analogue display output (VGA connector) to my analogue oscilloscope: Blue channel onto scope channel 1, green channel to scope channel 2, external triggering on line synchronization signal (HSync). A quick and dirty OpenGL program, deliberately written with immediate mode was used to generate a linear color ramp:

Either way the values you present to the scanout (which means the on-screen framebuffers) will undergo a nonlinear mapping at some point in the signal chain. And for all standard consumer devices this mapping will be according to the sRGB standard, because it"s the smallest common factor (i.e. images represented in the sRGB color space can be reproduced on most output devices).

Since most programs, like webbrowsers assume the output to undergo a sRGB to display color space mapping, they simply copy the pixel values of the standard image file formats to the on-screen frame as they are, without performing a color space conversion, thereby implying that the color values within those images are in sRGB color space (or they will often merely convert to sRGB, if the image color profile is not sRGB); the correct thing to do (if, and only if the color values written to the framebuffer are scanned out to the display unaltered; assuming that scanout LUT is part of the display), would be conversion to the specified color profile the display expects.

This is where the ARB_framebuffer_sRGB extension (which went core with OpenGL-3) enters the picture, which introduced new flags used for the configuration of window pixelformats:

So if you have a window configured with such a sRGB pixelformat and enable sRGB rasterization mode in OpenGL with glEnable(GL_FRAMEBUFFER_SRGB); the result of the linear colorspace rendering operations will be transformed in sRGB color space.

But that"s only the output side of rendering signal chain. You also got input signals, in the form of textures. And those are usually images, with their pixel values stored nonlinearly. So before those can be used in linear image operations, such images must be brought into a linear color space first. Lets just ignore for the time being, that mapping nonlinear color spaces into linear color spaces opens several of cans of worms upon itself – which is why the sRGB color space is so ridiculously small, namely to avoid those problems.

So to address this an extension EXT_texture_sRGB was introduced, which turned out to be so vital, that it never went through being ARB, but went straight into the OpenGL specification itself: Behold the GL_SRGB… internal texture formats.

A texture loaded with this format undergoes a sRGB to linear RGB colorspace transformation, before being used to source samples. This gives linear pixel values, suitable for linear rendering operations, and the result can then be validly transformed to sRGB when going to the main on-screen framebuffer.

What one really wants is to have the on-screen framebuffer in a linear, contact color space; the natural choice would be CIEXYZ. Rendering operations would naturally take place in the same contact color space. Doing all graphics operations in contact color spaces, avoids the opening of the aforementioned cans-of-worms involved with trying to push a square peg named linear RGB through a nonlinear, round hole named sRGB.

Yes, indeed. If somewhere in the signal chain a nonlinear transform is applied, but all the pixel values go unmodified from the image to the display, then that nonlinearity has already been pre-applied on the image"s pixel values. Which means, that the image is already in a nonlinear color space.

That"s indeed the case. Because color management was added to all the widespread graphics systems as an afterthought, most image editors edit pixel values in their destination color space. Note that one particular design parameter of sRGB was, that it should merely retroactively specify the unmanaged, direct value transfer color operations as they were (and mostly still are done) done on consumer devices. Since there happens no color management at all, the values contained in the images and manipulated in editors must be in sRGB already. This works for so long, as long images are not synthetically created in a linear rendering process; in case of the later the render system has to take into account the destination color space.

Gamma correction is a technique used to map linearly increasing brightness data to a display device in a way that conveys linearly increasing intensity. As displays are nonlinear devices, gamma correction requires a nonlinear adjustment to be made to brightness values before being sent to the display. Ideally, gamma corrected linear steps in the brightness of a pixel will result in linear steps in perceived intensity. The application in antialiasing is that high contrast edges can appear under aliased if the brightness of a pixel isn"t adjusted high enough for humans to perceive an increase in intensity after being displayed by the monitor.

Unfortunately, gamma correcting AA isn"t always desirable. Different CRT, LCD, and TVs have different gamma characteristics that make choosing one gamma correction scheme more or less effective per device. It can also result in brighter colored sub-samples having a heavier influence on the color of a pixel than darker sub-samples. This causes problems for thing like thin lines.

Really, edge AA with and without gamma correction is six of one and half a dozen of the other. Combine this with the fact that the effect is different depending on the monitor being used and the degraded visibility of thin lines and we feel that gamma correct AA isn"t a feature that improves image quality as much as it just changes it.

While we are happy that NVIDIA has given us the choice to enable or disable gamma correct AA as we see fit, with G80 the default state has changed to enabled. While this doesn"t have an impact on performance, we prefer rendering without gamma correct AA enabled and will do so in our performance tests. We hope that ATI will add a feature to disable gamma correct AA in the future as well. For now, let"s take a look at R580 and G80 compared with gamma correction enabled.

At 4xAA with gamma correction enabled, it looks like ATI is able to produce a better quality image. Some of the wires and antenna on NVIDIA hardware area a little more ragged looking while ATI"s images are smoothed better.

The image appears dark because it is in the linear RGB color space. Apply gamma correction to the image according to the sRGB standard, storing the values in double precision.

Display gamma is numerical expression that describes the relationship between signal input and video output of a display device. As you increase signal intensity, displays do not produce linear increases in light output. The relationship between signal input and video output is non-linear. To correct for this, reciprocal non-linearity is applied at the production stage. This is typically referred to as camera gamma. The combination of these two opposite nonlinear luminance curves—camera gamma at the production end and display gamma at the device end—results in a linear system gamma of 1.0, which is what we want. However, when viewing material in a dim environment, it is generally thought desirable to have a system gamma that is slightly higher: somewhere between 1.1 and 1.2 is most often quoted figure.

Assuming a Rec. 709 camera encode gamma of 0.51, this means that display gamma should be in the 2.2-2.35 range, but what does this mean? For an idea of what different display gammas provide, see the chart below.

These numbers are calculated by using a standard power law. Quite simply, the output at any given level is just that percentage of 100% video that is the level of input to the power of the gamma used. For example, if we assume a 2.2 gamma, then an 80% input results in an output that is 61.21% of 100% luminance, or 0.8^2.2. So to calculate the desired level of output at any video level, all you need to know is the measured luminance at 100% and the desired gamma.

As you can see, the various gammas all begin and end with a one-to-one relationship between input and output. Zero input produces zero output (actually, because of the display’s residual black level it is really just the minimum amount of light the display produces, not literally zero) and maximum input produces maximum output. This is as you would expect. However, the precise relationship between input and output as you gradually increase input from 0% and above is not linear, as shown above, and it varies depending on gamma.

A display with lower gamma increases its light output more quickly as you increase the signal input. If you look at the 10% input, you will see that a 2.8 gamma produces only 16% of the light output of 2.0 gamma. This is obviously an enormous difference. The difference becomes increasingly less significant as the input rises, so that at 80% input a display with a 2.8 gamma produces nearly 84% of the output of a display with 2.0 gamma, which would be barely noticeable.

For this reason, the primary effect of gamma on image quality will at the low end of the video scale impacting most obviously shadow detail and black levels. If the gamma is too low you will achieve great shadow detail but your black levels will be noticeably elevated and contrast will suffer. If you raise gamma too high then you will create deep, dark blacks but with compromised shadow detail.

There are several myths about how to properly set gamma. For example, Rec. 709—the high definition standard—includes an encoding specification, but no decoding specification, so it provides no help in setting display gamma properly.

sRGB—the standard for computer monitors and which incidentally includes exactly the same gamut and white point as Rec. 709—does have a complete gamma specification. It is sometimes claimed that sRGB recommends a display gamma of 2.2. This is not quite correct. Although the sRGB display gamma is on average near 2.2, it actually recommends a higher gamma at the top end of the video range and a much lower gamma at the bottom of the video range. In general, this is a good approach, but sRGB is intended for viewing conditions that are considerably brighter than what one experiences in the typical home theater environment where lighting tends to be low or even completely dark.

Others argue that one should use a display gamma of 2.4, especially if one has a high contrast display. This stems partially from the fact that Rec. 709 implies a 2.4 display gamma and many professional studio environments reportedly use 2.4 when mastering content for Blu-ray release. This is also not quite true. Calibrating a display to a straight power curve of 2.4 will only result in substantially reduced shadow detail and an unnatural "contrasty" quality to the image.

The correct approach is suggested by the sRGB standard and has fairly recently been codified in a new gamma specification called BT.1886, which uses 2.4 as a starting point but adjusts the overall response curve depending on the black level and white level of the display. Like sRGB, BT.1886 recommends a gamma response that is higher at the top end than at the low end. A straight power curve of 2.4 is correct only if the display has a zero black level and an infinite contrast ratio, which no real-world display has. The full BT.1886 specification is complex and its precise recommendations vary depending upon the white level, and especially the black level, of the display. However, if you don"t want to bother with a precise BT.1886 calculation, white/black values of 120/0.03 cd/m2 serve as a good rule of thumb. This results in a gamma response between 2.3-2.4 at the top end and 2.2-2.1 on the low end.

To develop specifications for a video calibration system, you not only need to know how to calculate gamma, but degamma as well. degamma is just the process of removing gamma. There are different formulas for degamma. For a straight power law gamma degamma is just the reciprocal of the gamma: 1/gamma. BT.1886 degamma assumes the reciprocal of 2.4. sRGB uses a more complicated formula for removing gamma, but in practice it ends up being almost identical to the reciprocal of 2.2.

Degamma is important for two reasons. First, the colors we typically start with are specified in xyY, which has gamma by definition. We will want to know the linear RGB equivalent of that xyY color. To calculate that we need to convert the xyY color to R’G’B’, which is gamma-weighted RGB and then remove gamma to get linear RGB. Unlike most color spaces, RGB has a non-linear version that includes gamma and a linear version that does not. Linear RGB is important for a number of reasons, but the most obvious is that it forms the basis of test patterns. To know the 8-bit (16-235) or 10-bit (64-940) test pattern for a specific xyY color, you have to calculate the linear RGB value of that color, from which the RGB triplet test pattern is derived.

HDR gamma works very differently from all of the other gamma systems discussed above. HDR10—which is currently the dominant standard, though several others are waiting in the wings—assumes that 100% output is an absolute value of 10,000 cd/m2. This is unlike any other gamma system for which 100% is a relative value that changes depending upon the capabilities of the display. The absolute spec of 10,000 cd/m2 causes all sorts of problems for HDR10, not the least of which is that it becomes impossible to calibrate gamma above 60%-75% video input. Current displays are simply not capable of outputting 10,000 cd/m2, so all the user can do is measure and calibrate up to the maximum video level that HDR10 allows, and for current displays this is generally no more than 75%. Above that you just have to let the display clip. Some people talk about tone mapping, which would gradually roll off the response instead of abruptly clipping at the limit of the display’s ability. However, there is currently no standard for this.

There are several points to keep in mind about gamma: The gamma of an HD display should be either a power law 2.22, sRGB, or BT.1886. BT.1886 is the preferred standard. The gamma of a UHD display showing UHD content should be HDR.

The gamma of red, green, and blue, should all be the same. If they are different, then you will have a problem with grayscale tracking. In fact, grayscale tracking and RGB gamma are essentially the same.

Do not use the contrast and brightness controls to affect gamma. Use a pluge pattern to set brightness and a white pluge