wave and particle behaviors of lcd displays made in china

The theoretical sketch of the wave-particle scheme for the single photon is displayed in Fig. 1. A photon is initially prepared in a polarization state \(\left| {{\psi _0}} \right\rangle = {\rm{cos}}\,\alpha \left| {\rm V} \right\rangle + {\rm{sin}}\,\alpha \left| {\rm{H}} \right\rangle\), where \(\left| {\rm V} \right\rangle\) and \(\left| {\rm{H}} \right\rangle\) are the vertical and horizontal polarization states and α is adjustable by a preparation half-wave plate (not shown in the figure). After crossing the preparation part of the setup of Fig. 1 (see Supplementary Notes 1 and 2 and Supplementary Fig. 1 for details), the photon state is

$$\left| {{\psi _{\rm{f}}}} \right\rangle = {\rm{cos}}\,\alpha \left| {{\rm{wave}}} \right\rangle + {\rm{sin}}\,\alpha \left| {{\rm{particle}}} \right\rangle ,$$

$$\begin{array}{*{20}{l}}\\ {\left| {{\rm{wave}}} \right\rangle } = {{e^{i{\phi _1}/2}}\left( {{\rm{cos}}\frac{{{\phi _1}}}{2}\left| 1 \right\rangle - i\,{\rm{sin}}\frac{{{\phi _1}}}{2}\left| 3 \right\rangle } \right),} \hfill \\ {\left| {{\rm{particle}}} \right\rangle } = {\frac{1}{{\sqrt 2 }}\left( {\left| 2 \right\rangle + {e^{i{\phi _2}}}\left| 4 \right\rangle } \right),} \hfill \\ \end{array}$$

operationally represent the capacity \(\left( {\left| {{\rm{wave}}} \right\rangle } \right)\) and incapacity \(\left( {\left| {{\rm{particle}}} \right\rangle } \right)\) of the photon to produce interference\(\left| {{\rm{wave}}} \right\rangle\) state the probability of detecting the photon in the path \(\left| n \right\rangle \,\) (n = 1, 3) depends on the phase ϕ

1: the photon must have traveled along both paths simultaneously (see upper MZI in Fig. 1), revealing its wave behavior. Instead, for the \(\left| {{\rm{particle}}} \right\rangle\) state the probability to detect the photon in the path \(\left| n \right\rangle \,\) (n = 2, 4) is 1/2, regardless of phase ϕ

2: thus, the photon must have crossed only one of the two paths (see lower MZI of Fig. 1), showing its particle behavior. Notice that the scheme is designed in such a way that \(\left| {\rm V} \right\rangle\)

Conceptual figure of the wave-particle toolbox. A single photon is coherently separated in two spatial modes by means of a polarizing beam-splitter (PBS) according to its initial polarization state (in). A half-wave plate (HWP) is placed after the PBS to obtain equal polarizations between the two modes. One mode is injected in a complete Mach-Zehnder interferometer (MZI) with phase ϕ

1, thus exhibiting wave-like behavior. The second mode is injected in a Mach-Zehnder interferometer lacking the second beam-splitter, thus exhibiting particle-like behavior (no dependence on ϕ

To verify the coherent wave-particle superposition as a function of the parameter α, the wave and particle states have to interfere at the detection level. This goal is achieved by exploiting two symmetric beam-splitters where the output paths (modes) are recombined, as illustrated in the detection part of Fig. 1. The probability P

We remark that the terms \({{\cal I}_{\rm{c}}}\), \({{\cal I}_{\rm{s}}}\) in the detection probabilities exclusively stem from the interference between the \(\left| {{\rm{wave}}} \right\rangle\) and \(\left| {{\rm{particle}}} \right\rangle\) components appearing in the generated superposition state \(\left| {{\psi _{\rm{f}}}} \right\rangle\) of Eq. (1). This fact is further evidenced by the appearance, in these interference terms, of the factor \({\cal C} = {\rm{sin}}\,2\alpha\), which is the amount of quantum coherence owned by \(\left| {{\psi _{\rm{f}}}} \right\rangle\) in the basis {\(\left| {{\rm{wave}}} \right\rangle\), \(\left| {{\rm{particle}}} \right\rangle\)} theoretically quantified according to the standard l

1-norm\({{\cal I}_{\rm{c}}}\), \({{\cal I}_{\rm{s}}}\) are always identically zero (independently of phase values) when the final state of the photon is: (i) \(\left| {{\rm{wave}}} \right\rangle\) (α = 0); (ii) \(\left| {{\rm{particle}}} \right\rangle\) (α = π/2); (iii) a classical incoherent mixture \({\rho _{\rm{f}}} = {\rm{co}}{{\rm{s}}^2}\alpha \left| {{\rm{wave}}} \right\rangle \left\langle {{\rm{wave}}} \right| + {\rm{si}}{{\rm{n}}^2}\alpha \left| {{\rm{particle}}} \right\rangle \left\langle {{\rm{particle}}} \right|\) (which can be theoretically produced by the same scheme starting from an initial mixed polarization state of the photon).

The experimental single-photon toolbox, realizing the proposed scheme of Fig. 1, is displayed in Fig. 2 (see Methods for more details). The implemented layout presents the advantage of being interferometrically stable, thus not requiring active phase stabilization between the modes. Figure 3 shows the experimental results for the measured single-photon probabilities P

n. For α = 0, the photon is vertically polarized and entirely reflected from the PBS to travel along path 1, then split at BS1 into two paths, both leading to the same BS3 which allows these two paths to interfere with each other before detection. The photon detection probability at each detector Dn (n = 1, 2, 3, 4) depends on the phase shift ϕ

1: \({P_1}\left( {\alpha = 0} \right) = {P_2}\left( {\alpha = 0} \right) = \frac{1}{2}{\rm{co}}{{\rm{s}}^2}\frac{{{\phi _1}}}{2}\), \({P_3}\left( {\alpha = 0} \right) = {P_4}\left( {\alpha = 0} \right) = \frac{1}{2}{\rm{si}}{{\rm{n}}^2}\frac{{{\phi _1}}}{2}\), as expected from Eqs. (3) and (4). After many such runs an interference pattern emerges, exhibiting the wave-like nature of the photon. Differently, if initially α = π/2, the photon is horizontally polarized and, as a whole, transmitted by the PBS to path 2, then split at BS2 into two paths (leading, respectively, to BS4 and BS5) which do not interfere anywhere. Hence, the phase shift ϕ

2 plays no role on the photon detection probability and each detector has an equal chance to click: \({P_1}\left( {\alpha = \frac{\pi }{2}} \right) = {P_2}\left( {\alpha = \frac{\pi }{2}} \right) = {P_3}\left( {\alpha = \frac{\pi }{2}} \right) = {P_4}\left( {\alpha = \frac{\pi }{2}} \right) = \frac{1}{4}\), as predicted by Eqs. (3) and (4), showing particle-like behavior without any interference pattern. Interestingly, for 0 < α < π/2, the photon simultaneously behaves like wave and particle. The coherent continuous morphing transition from wave to particle behavior as α varies from 0 to π/2 is clearly seen from Fig. 4a and contrasted with the morphing observed for a mixed incoherent wave-particle state ρ

2 = 0, the coherence of the generated state is also directly quantified by measuring the expectation value of an observable \(\sigma _x^{1234}\), defined in the four-dimensional basis of the photon paths \(\left\{ {\left| 1 \right\rangle ,\left| 2 \right\rangle ,\left| 3 \right\rangle ,\left| 4 \right\rangle } \right\}\) of the preparation part of the setup as a Pauli matrix σ

x between modes (1, 2) and between modes (3, 4). It is then possible to straightforwardly show that \(\left\langle {\sigma _x^{1234}} \right\rangle = {\rm{Tr}}\left( {\sigma _x^{1234}{\rho _{\rm{f}}}} \right) = 0\) for any incoherent state ρ

f, while \(\sqrt 2 \left\langle {\sigma _x^{1234}} \right\rangle = {\rm{sin}}\,2\alpha = {\cal C}\) for an arbitrary state of the form \(\left| {{\psi _{\rm{f}}}} \right\rangle\) defined in Eq. (1). Insertion of beam-splitters BS4 and BS5 in the detection part of the setup (corresponding to β = 22.5° in the output wave-plate of Fig. 2) rotates the initial basis \(\left\{ {\left| 1 \right\rangle ,\left| 2 \right\rangle ,\left| 3 \right\rangle ,\left| 4 \right\rangle } \right\}\) generating a measurement basis of eigenstates of \(\sigma _x^{1234}\), whose expectation value is thus obtained in terms of the detection probabilities as \(\left\langle {\sigma _x^{1234}} \right\rangle = {P_1} - {P_2} + {P_3} - {P_4}\) (see Supplementary Note 2). As shown in Fig. 4c, d, the observed behavior of \(\sqrt 2 \left\langle {\sigma _x^{1234}} \right\rangle\) as a function of α confirms the theoretical predictions for both coherent \(\left| {{\psi _{\rm{f}}}} \right\rangle\) (Fig. 4c) and mixed (incoherent) ρ

f wave-particle states (the latter being obtained in the experiment by adding a relative time delay in the interferometer paths larger than the photon coherence time to lose quantum interference, Fig. 4d).

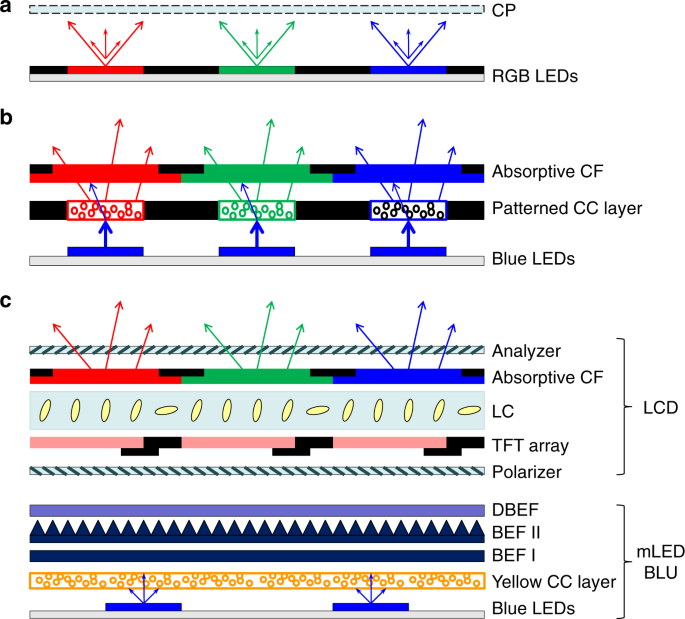
Experimental setup for wave-particle states. a Overview of the apparatus for the generation of single-photon wave-particle superposition. An heralded single-photon is prepared in an arbitrary linear polarization state through a half-wave plate rotated at an angle α/2 and injected into the wave-particle toolbox. b Overview of the apparatus for the generation of a two-photon wave-particle entangled state. Each photon of a polarization entangled state is injected into an independent wave-particle toolbox to prepare the output state. c Actual implemented wave-particle toolbox, reproducing the action of the scheme shown in Fig. 1. Top subpanel: top view of the scheme, where red and purple lines represent optical paths lying in two vertical planes. Bottom subpanel: 3-d scheme of the apparatus. The interferometer is composed of beam-displacing prisms (BDP), half-wave plates (HWP), and liquid crystal devices (LC), the latter changing the phases ϕ

2. The output modes are finally separated by means of a polarizing beam-splitter (PBS). The scheme corresponds to the presence of BS4 and BS5 in Fig. 1 for β = 22.5°, while setting β = 0 equals to the absence of BS4 and BS5. The same color code for the optical elements (reported in the figure legend) is employed for the top view and for the 3-d view of the apparatus. d Picture of the experimental apparatus. The green frame highlights the wave-particle toolbox

1, for different values of α. a Wave behavior (α = 0). b Particle behavior (α = π/2). c Coherent wave-particle superposition (α = π/4). d Incoherent mixture of wave and particle behaviors (α = π/4). Points: experimental data. Dashed curves: best-fit of the experimental data. Color legend: orange (P1), green (P2), purple (P3), blue (P

1 and of the angle α. Points: experimental data. Surfaces: theoretical expectations. In all plots, error bars are standard deviation due to the Poissonian statistics of single-photon counting

4). In a black triangles highlighted the position for wave behavior (α = 0), black circle for particle behavior (α = π/2) and black diamonds highlight the position for coherent wave-particle superposition behavior (α = π/4). c Coherence measure \(\sqrt 2 \left\langle {\sigma _x^{1234}} \right\rangle\) as a function of α in the coherent case and d for an incoherent mixture (the latter showing no dependence on α). Points: experimental data. Solid curves: theoretical expectations. Error bars are standard deviations due to the Poissonian statistics of single-photon counting

The above single-photon scheme constitutes the basic toolbox which can be extended to create a wave-particle entangled state of two photons, as shown in Fig. 2b. Initially, a two-photon polarization maximally entangled state \({\left| \Psi \right\rangle _{{\rm{AB}}}} = \frac{1}{{\sqrt 2 }}\left( {\left| {{\rm VV}} \right\rangle + \left| {{\rm{HH}}} \right\rangle } \right)\) is prepared (the procedure works in general for arbitrary weights, see Supplementary Note 3). Each photon is then sent to one of two identical wave-particle toolboxes which provide the final state

$${\left| \Phi \right\rangle _{{\rm{AB}}}} = \frac{1}{{\sqrt 2 }}\left( {\left| {{\rm{wave}}} \right\rangle \left| {{\rm{wave}"}} \right\rangle + \left| {{\rm{particle}}} \right\rangle \left| {{\rm{particle}"}} \right\rangle } \right),$$

where the single-photon states \(\left| {{\rm{wave}}} \right\rangle\), \(\left| {{\rm{particle}}} \right\rangle\), \(\left| {{\rm{wave}"}} \right\rangle\), \(\left| {{\rm{particle}"}} \right\rangle\) are defined in Eq. (2), with parameters and paths related to the corresponding wave-particle toolbox. Using the standard concurrenceC to quantify the amount of entanglement of this state in the two-photon wave-particle basis, one immediately finds C = 1. The generated state \({\left| \Phi \right\rangle _{{\rm{AB}}}}\) is thus a wave-particle maximally entangled state (Bell state) of two photons in separated locations.

The output two-photon state is measured after the two toolboxes. The results are shown in Fig. 5. Coincidences between the four outputs of each toolbox are measured by varying ϕ

1 and \(\phi _1^{\prime}\). The first set of measurements (Fig. 5a–d) is performed by setting the angles of the output wave-plates (see Fig. 2c) at {β = 0, β′ = 0}, corresponding to removing both BS4 and BS5 in Fig. 1 (absence of interference between single-photon wave and particle states). In this case, detectors placed at outputs (1, 3) and (1′, 3′) reveal wave-like behavior, while detectors placed at outputs (2, 4) and (2′, 4′) evidence a particle-like one. As expected, the two-photon probabilities \({P_{n{n^{\prime}}}}\) for the particle detectors remain unchanged while varying ϕ

1 and \(\phi _1^{\prime}\), whereas the \({P_{nn"}}\) for the wave detectors show interference fringes. Moreover, no contribution of crossed wave-particle coincidences \({P_{nn"}}\) is obtained, due to the form of the entangled state. The second set of measurements (Fig. 5e–h) is performed by setting the angles of the output wave-plates at {β = 22.5°, β′ = 22.5°}, corresponding to the presence of BS4 and BS5 in Fig. 1 (the presence of interference between single-photon wave and particle states). We now observe nonzero contributions across all the probabilities depending on the specific settings of phases ϕ

1 and \(\phi _1^{\prime}\). The presence of entanglement in the wave-particle behavior is also assessed by measuring the quantity \({\cal E} = {P_{22"}} - {P_{21"}}\) as a function of ϕ

1, with fixed \(\phi _1^{\prime} = {\phi _2} = \phi _2^{\prime} = 0\). According to the general expressions of the coincidence probabilities (see Supplementary Note 3), \({\cal E}\) is proportional to the concurrence C and identically zero (independently of phase values) if and only if the wave-particle two-photon state is separable (e.g., \(\left| {{\rm{wave}}} \right\rangle\) ⊗ \(\left| {{\rm{wave}"}} \right\rangle\) or a maximal mixture of two-photon wave and particle states). For \(\left| \Phi \right\rangle\)

AB of Eq. (5) the theoretical prediction is \({\cal E} = \left( {1{\rm{/}}4} \right){\rm{co}}{{\rm{s}}^{\rm{2}}}\left( {{\phi _1}{\rm{/}}2} \right)\), which is confirmed by the results reported in Fig. 5i, j (within the reduction due to visibility). A further test of the generated wave-particle entanglement is finally performed by the direct measure of the expectation values \(\left\langle {\cal W} \right\rangle = {\rm{Tr}}\left( {{\cal W}\rho } \right)\) of a suitable entanglement witness

z Pauli matrix between modes (1, 2) and between modes (3, 4). The measurement basis of \(\sigma _z^{1234}\) is that of the initial paths \(\left\{ {\left| 1 \right\rangle ,\left| 2 \right\rangle ,\left| 3 \right\rangle ,\left| 4 \right\rangle } \right\}\) exiting the preparation part of the single-photon toolbox. It is possible to show that \({\rm{Tr}}\left( {{\cal W}{\rho _{\rm{s}}}} \right) \ge 0\) for any two-photon separable state ρ

e is entangled in the photons wave-particle behavior (see Supplementary Note 3). The expectation values of \({\cal W}\) measured in the experiment in terms of the 16 coincidence probabilities P

1 = 0, \(\phi _1^\prime\) = π). These observations altogether prove the existence of quantum correlations between wave and particle states of two photons in the entangled state \(\left| \Phi \right\rangle\)

Generation of wave-particle entangled superposition with a two-photon state. Measurements of the output coincidence probabilities \({P_{nn"}}\) to detect one photon in output mode n of the first toolbox and one in the output mode n′ of the second toolbox, with different phases ϕ

1 = π and \(\phi _1^{\prime} = \pi\). White bars: theoretical predictions. Colored bars: experimental data. Orange bars: \({P_{n{n^{\prime}}}}\) contributions for detectors Dn and \({{\rm{D}}_{n"}}\) linked to wave-like behavior for both photons (in the absence of BS4 and BS5). Cyan bars: \({P_{nn"}}\) contributions for detectors Dn and \({{\rm{D}}_{n"}}\) linked to particle-like behavior for both photons (in the absence of BS4 and BS5). Magenta bars: \({P_{nn"}}\) contributions for detectors Dn and \({{\rm{D}}_{n"}}\) linked to wave-like behavior for one photon and particle-like behavior for the other one (in absence of BS4 and BS5). Darker regions in colored bars correspond to 1 σ error interval, due to the Poissonian statistics of two-photon coincidences. i, j, Quantitative verification of wave-particle entanglement. i, \({P_{22"}}\) (blue) and \({P_{21"}}\) (green) and j, \({\cal E} = {P_{22"}} - {P_{21"}}\), as a function of ϕ

1 for \(\phi _1^{\prime} = 0\) and {β = 22.5°, β′ = 22.5°}. Error bars are standard deviations due to the Poissonian statistics of two-photon coincidences. Dashed curves: best-fit of the experimental data

China"s first 8.5-generation TFT-LCD production line was launched in Bengbu, East China"s Anhui province, on June 18, 2019, representing a breakthrough in the production of high-definition LCD screen, Science and Technology Daily reported.

TFT-LCD, or Thin Film Transistor Liquid Crystal Display, is key strategic material of the electronic information display industry. The Gen 8.5 TFT-LCD production line, launched by the Bengbu Glass Industry Design and Research Institute of the China National Building Material Group, will produce high-definition LCD screens of 55 inches, the report said.

According to the Liquid Crystal Branch of the China Optics and Optoelectronics Manufactures Association, the demand for TFT-LCD in the Chinese mainland was about 260 million square meters in 2018, including 233 million square meters" Gen 8.5 TFT-LCD. However, the annual supply of domestically made TFT-LCD is less than 40 million square meters, with all of them Gen 6 or below, which cannot meet the demand in scale and quantity.

The association predicted that China"s market demand for Gen 8.5 TFT-LCD or above will exceed 300 million square meters by 2020, accounting for 49.6 percent of the total global demand.

The production and control precision of Gen 8.5 TFT-LCD is comparable to that of the semiconductor industry, representing a higher level of large-scale manufacturing of modern glass industry.

The institute in Bengbu, with 60 years of expertise in glass, has finally made a breakthrough in the production of Gen 8.5 TFT-LCD, and will provide key raw material guarantee for China"s LCD panel industry after it goes into mass production in September, the report said.

The double-slit experiment is one of the most famous experiments in physics and definitely one of the weirdest. It demonstrates that matter and energy (such as light) can exhibit both wave and particle characteristics — known as the particle-wave duality of matter — depending on the scenario, according to the scientific communication site Interesting Engineering(opens in new tab).

According to the University of Sussex(opens in new tab), American physicist Richard Feynman referred to this paradox as the central mystery of quantum mechanics.

Christian Huygens was the first to describe light as traveling in waves whilst Isaac Newton thought light was composed of tiny particles according to Las Cumbres Observatory(opens in new tab). But who is right? British polymath Thomas Young designed the double-slit experiment to put these theories to the test.

To appreciate the truly bizarre nature of the double-split experiment we first need to understand how waves and particles act when passing through two slits.

Firstly, if we were to shine a light on a wall with two parallel slits — and for the sake of simplicity, let"s say this light has only one wavelength.

According to Stony Brook University(opens in new tab), any wave can create an interference pattern, whether it be a sound wave, light wave or waves across a body of water. When a wave crest hits a wave trough they cancel each other out — known as destructive interference — and appear as a dark band. When a crest hits a crest they amplify each other — known as constructive interference — and appear as a bright band. The combination of dark and bright bands is known as an interference pattern and can be seen on the sensor screen opposite the slits.

But that is not the whole story. Light is a little more complicated than that, and to see how strange it really is we also need to understand what pattern a particle would make on a sensor field.

Clearly, waves and particles produce a very different pattern, so it should be easy to distinguish between the two right? Well, this is where the double-slit experiment gets a little strange when we try and carry out the same experiment but with tiny particles of light called photons. Enter the realm of quantum mechanics.

The smallest constituent of light is subatomic particles called photons. By using photons instead of grains of sand we can carry out the double-slit experiment on an atomic scale.

If you unblock the slit and fire photons through both slits, you start to see something very similar to the interference pattern produced by waves in the light example. The photons appear to have gone through the pair of slits acting like waves.

But what if you launch photons one by one, leaving enough time between them that they don"t have a chance of interfering with each other, will they behave like particles or waves?

It"s almost as though each photon is "aware" that there are two slits available. How? Does it split into two and then rejoin after the slit and then hit the sensor? To investigate this, scientists set up a detector that can tell which slit the photon passes through.

Again, we fire photons one at a time at the slits, as we did in the previous example. The detector finds that about 50% of the photons have passed through the top slit and about 50% through the bottom, and confirms that each photon goes through one slit or the other. Nothing too unusual there.

This pattern matches the one we saw when we fired particles through the slits. It appears that monitoring the photons triggers them to switch from the interference pattern produced by waves to that produced by particles.

If the detection of photons through the slits is apparently affecting the pattern on the sensor screen, what happens if we leave the detector in place but switch it off? (Shh, don"t tell the photons we"re no longer spying on them!)

The atoms appear to act like waves when you"re not watching them, but as particles when you are. How? Well, if you can answer that, a Nobel Prize is waiting for you.

In the 1930s, scientists proposed that human consciousness might affect quantum mechanics. Mathematician John Von Neumann first postulated this in 1932 in his book "The Mathematical Foundations of Quantum Mechanics." In the 1960s, theoretical physicist, Eugene Wigner conceived a thought experiment called Wigner"s friend — a paradox in quantum physics that describes the states of two people, one conducting the experiment and the observer of the first person, according to science magazine Popular Mechanics. The idea that the consciousness of a person carrying out the experiment can affect the result is knowns as the Von Neumann–Wigner interpretation.

Though a spiritual explanation for quantum mechanic behavior is still believed by a few individuals, including author and alternative medicine advocate Deepak Chopra, a majority of the science community has long disregarded it.

Furthermore —and perhaps even more astonishingly — if you set up the double-slit experiment to detect which slit the photon went through after the photon has already hit the sensor screen, you still end up with a particle-type pattern on the sensor screen, even though the photon hadn"t yet been detected when it hit the screen. This result suggests that detecting a photon in the future affects the pattern produced by the photon on the sensor screen in the past. This experiment is known as the quantum eraser experiment and is explained in more detail in this informative video from Fermilab(opens in new tab).

We still don"t fully understand how exactly the particle-wave duality of matter works, which is why it is regarded as one of the greatest mysteries of quantum mechanics.

The first version of the double-slit experiment was carried out in 1801 by British polymath Thomas Young, according to the American Physical Society(opens in new tab) (APS). His experiment demonstrated the interference of light waves and provided evidence that light was a wave, not a particle.

Despite his convincing experiment that light was a wave, those who did not want to accept that Isaac Newton could have been wrong about something criticized Young. (Newton had proposed the corpuscular theory, which posited that light was composed of a stream of tiny particles he called corpuscles.)

According to APS, Young wrote in response to one of the critics, "Much as I venerate the name of Newton, I am not therefore obliged to believe that he was infallible."

Explore the double-slit experiment in more detail with this article from the University of Cambridge,(opens in new tab)which includes images of electron patterns in a double-slit experiment. Discover the true nature of light with Canon Science Lab(opens in new tab). Read about fragments of energy that are not waves or particles — but could be the fundamental building blocks of the universe — in this article from The Conversation(opens in new tab). Dive deeper into the two-slit experiment in this article published in the journal Nature(opens in new tab).

Thorn, J. J., et al. "Observing the quantum behavior of light in an undergraduate laboratory.(opens in new tab)" American Journal of Physics 72.9 (2004): 1210-1219.

Aharonov, Yakir, et al. "Finally making sense of the double-slit experiment.(opens in new tab)" Proceedings of the National Academy of Sciences 114.25 (2017): 6480-6485.

A cathode-ray tube (CRT) is a vacuum tube containing one or more electron guns, which emit electron beams that are manipulated to display images on a phosphorescent screen.waveforms (oscilloscope), pictures (television set, computer monitor), radar targets, or other phenomena. A CRT on a television set is commonly called a picture tube. CRTs have also been used as memory devices, in which case the screen is not intended to be visible to an observer. The term

In CRT television sets and computer monitors, the entire front area of the tube is scanned repeatedly and systematically in a fixed pattern called a raster. In color devices, an image is produced by controlling the intensity of each of three electron beams, one for each additive primary color (red, green, and blue) with a video signal as a reference.magnetic deflection, using a deflection yoke. Electrostatic deflection is commonly used in oscilloscopes.

A CRT is a glass envelope which is deep (i.e., long from front screen face to rear end), heavy, and fragile. The interior is evacuated to 0.01 pascals (1×10−7 atm)×10−12 atm) or less,implosion that can hurl glass at great velocity. The face is typically made of thick lead glass or special barium-strontium glass to be shatter-resistant and to block most X-ray emissions. CRTs make up most of the weight of CRT TVs and computer monitors.

Since the mid-late 2000"s, CRTs have been superseded by flat-panel display technologies such as LCD, plasma display, and OLED displays which are cheaper to manufacture and run, as well as significantly lighter and less bulky. Flat-panel displays can also be made in very large sizes whereas 40 in (100 cm) to 45 in (110 cm)

Cathode rays were discovered by Julius Plücker and Johann Wilhelm Hittorf.cathode (negative electrode) which could cast shadows on the glowing wall of the tube, indicating the rays were traveling in straight lines. In 1890, Arthur Schuster demonstrated cathode rays could be deflected by electric fields, and William Crookes showed they could be deflected by magnetic fields. In 1897, J. J. Thomson succeeded in measuring the charge-mass-ratio of cathode rays, showing that they consisted of negatively charged particles smaller than atoms, the first "subatomic particles", which had already been named George Johnstone Stoney in 1891. The earliest version of the CRT was known as the "Braun tube", invented by the German physicist Ferdinand Braun in 1897.cold-cathode diode, a modification of the Crookes tube with a phosphor-coated screen. Braun was the first to conceive the use of a CRT as a display device.

The first cathode-ray tube to use a hot cathode was developed by John Bertrand Johnson (who gave his name to the term Johnson noise) and Harry Weiner Weinhart of Western Electric, and became a commercial product in 1922.

In the 1930s, Allen B. DuMont made the first CRTs to last 1,000 hours of use, which was one of the factors that led to the widespread adoption of television.

1968 marks the release of Sony Trinitron brand with the model KV-1310, which was based on Aperture Grille technology. It was acclaimed to have improved the output brightness. The Trinitron screen was identical with its upright cylindrical shape due to its unique triple cathode single gun construction.

In 1987, flat-screen CRTs were developed by Zenith for computer monitors, reducing reflections and helping increase image contrast and brightness.float glass.

In the mid-2000s, Canon and Sony presented the surface-conduction electron-emitter display and field-emission displays, respectively. They both were flat-panel displays that had one (SED) or several (FED) electron emitters per subpixel in place of electron guns. The electron emitters were placed on a sheet of glass and the electrons were accelerated to a nearby sheet of glass with phosphors using an anode voltage. The electrons were not focused, making each subpixel essentially a flood beam CRT. They were never put into mass production as LCD technology was significantly cheaper, eliminating the market for such displays.

Beginning in the late 90s to the early 2000s, CRTs began to be replaced with LCDs, starting first with computer monitors smaller than 15 inches in size,Hitachi in 2001,Flat-panel displays dropped in price and started significantly displacing cathode-ray tubes in the 2000s. LCD monitor sales began exceeding those of CRTs in 2003–2004

Despite being a mainstay of display technology for decades, CRT-based computer monitors and televisions are now virtually a dead technology. Demand for CRT screens dropped in the late 2000s.

Some industries still use CRTs because it is either too much effort, downtime, and/or cost to replace them, or there is no substitute available; a notable example is the airline industry. Planes such as the Boeing 747-400 and the Airbus A320 used CRT instruments in their glass cockpits instead of mechanical instruments.Lufthansa still use CRT technology, which also uses floppy disks for navigation updates.

A popular consumer usage of CRTs is for retrogaming. Some games are impossible to play without CRT display hardware, and some games play better. Reasons for this include:

The size of the screen of a CRT is measured in two ways: the size of the screen or the face diagonal, and the viewable image size/area or viewable screen diagonal, which is the part of the screen with phosphor. The size of the screen is the viewable image size plus its black edges which are not coated with phosphor.

Small CRTs below 3 inches were made for handheld televisions such as the MTV-1 and viewfinders in camcorders. In these, there may be no black edges, that are however truly flat.

Most of the weight of a CRT comes from the thick glass screen, which comprises 65% of the total weight of a CRT. The funnel and neck glass comprise the remaining 30% and 5% respectively. The glass in the funnel is thinner than on the screen.

The outer conductive coating is connected to ground while the inner conductive coating is connected using the anode button/cap through a series of capacitors and diodes (a Cockcroft–Walton generator) to the high voltage flyback transformer; the inner coating is the anode of the CRT,voltage multiplier for the current delivered by the flyback.

The anode is used to accelerate the electrons towards the screen and also collects the secondary electrons that are emitted by the phosphor particles in the vacuum of the CRT.

The anode cap connection in modern CRTs must be able to handle up to 55–60 kV depending on the size and brightness of the CRT. Higher voltages allow for larger CRTs, higher image brightness, or a tradeoff between the two.corona discharge.

The anode button must be specially shaped to establish a hermetic seal between the button and funnel. X-rays may leak through the anode button, although that may not be the case in newer CRTs starting from the late 1970s to early 1980s, thanks to a new button and clip design.

The flyback transformer is also known as an IHVT (Integrated High Voltage Transformer) if it includes a voltage multiplier. The flyback uses a ceramic or powdered iron core to enable efficient operation at high frequencies. The flyback contains one primary and many secondary windings that provide several different voltages. The main secondary winding supplies the voltage multiplier with voltage pulses to ultimately supply the CRT with the high anode voltage it uses, while the remaining windings supply the CRT"s filament voltage, keying pulses, focus voltage and voltages derived from the scan raster. When the transformer is turned off, the flyback"s magnetic field quickly collapses which induces high voltage in its windings. The speed at which the magnetic field collapses determines the voltage that is induced, so the voltage increases alongside its speed. A capacitor (Retrace Timing Capacitor) or series of capacitors (to provide redundancy) is used to slow the collapse of the magnetic field.

The design of the high voltage power supply in a product using a CRT has an influence in the amount of x-rays emitted by the CRT. The amount of emitted x-rays increases with both higher voltages and currents. If the product such as a TV set uses an unregulated high voltage power supply, meaning that anode and focus voltage go down with increasing electron current when displaying a bright image, the amount of emitted x-rays is as its highest when the CRT is displaying a moderately bright images, since when displaying dark or bright images, the higher anode voltage counteracts the lower electron beam current and vice versa respectively. The high voltage regulator and rectifier vacuum tubes in some old CRT TV sets may also emit x-rays.

The electron gun emits the electrons that ultimately hit the phosphors on the screen of the CRT. The electron gun contains a heater, which heats a cathode, which generates electrons that, using grids, are focused and ultimately accelerated into the screen of the CRT. The acceleration occurs in conjunction with the inner aluminum or aquadag coating of the CRT. The electron gun is positioned so that it aims at the center of the screen.

It has a hot cathode that is heated by a tungsten filament heating element; the heater may draw 0.5 to 2 A of current depending on the CRT. The voltage applied to the heater can affect the life of the CRT.

There are several shortcircuits that can occur in a CRT electron gun. One is a heater-to-cathode short, that causes the cathode to permanently emit electrons which may cause an image with a bright red, green or blue tint with retrace lines, depending on the cathode (s) affected. Alternatively, the cathode may short to the control grid, possibly causing similar effects, or, the control grid and screen grid (G2)sputtering.

Since it is a hot cathode, it is prone to cathode poisoning, which is the formation of a positive ion layer that prevents the cathode from emitting electrons, reducing image brightness significantly or completely and causing focus and intensity to be affected by the frequency of the video signal preventing detailed images from being displayed by the CRT. The positive ions come from leftover air molecules inside the CRT or from the cathode itself

The amount of electrons generated by the cathodes is related to their surface area. A cathode with more surface area creates more electrons, in a larger electron cloud, which makes focusing the electron cloud into an electron beam more difficult.

The second (screen) grid of the gun (G2) accelerates the electrons towards the screen using several hundred DC volts. A negative currentWehnelt cylinder.

However, electrostatic focusing cannot be accomplished near the final anode of the CRT due to its high voltage in the dozens of Kilovolts, so a high voltage (≈600

There is a voltage called cutoff voltage which is the voltage that creates black on the screen since it causes the image on the screen created by the electron beam to disappear, the voltage is applied to G1. In a color CRT with three guns, the guns have different cutoff voltages. Many CRTs share grid G1 and G2 across all three guns, increasing image brightness and simplifying adjustment since on such CRTs there is a single cutoff voltage for all three guns (since G1 is shared across all guns).

During retracing of the electron beam, the preamplifier that feeds the video amplifier is disabled and the video amplifier is biased to a voltage higher than the cutoff voltage to prevent retrace lines from showing, or G1 can have a large negative voltage applied to it to prevent electrons from getting out of the cathode.Vertical blanking interval and Horizontal blanking interval.) Incorrect biasing can lead to visible retrace lines on one or more colors, creating retrace lines that are tinted or white (for example, tinted red if the red color is affected, tinted magenta if the red and blue colors are affected, and white if all colors are affected).

The electron beam may be affected by the earth"s magnetic field, causing it to normally enter the focusing lens off-center; this can be corrected using astigmation controls. Astigmation controls are both magnetic and electronic (dynamic); magnetic does most of the work while electronic is used for fine adjustments.

Some electron guns have a quadrupole lens with dynamic focus to alter the shape and adjust the focus of the electron beam, varying the focus voltage depending on the position of the electron beam to maintain image sharpness across the entire screen, specially at the corners.

The electron guns in color CRTs are driven by a video amplifier which takes a signal per color channel and amplifies it to 40-170v per channel, to be fed into the electron gun"s cathodes;

CRTs have a pronounced triode characteristic, which results in significant gamma (a nonlinear relationship in an electron gun between applied video voltage and beam intensity).

There are two types of deflection: magnetic and electrostatic. Magnetic is usually used in TVs and monitors as it allows for higher deflection angles (and hence shallower CRTs) and deflection power (which allows for higher electron beam current and hence brighter images)

Those that use magnetic deflection may use a yoke that has two pairs of deflection coils; one pair for vertical, and another for horizontal deflection.

The deflection coils are driven by sawtooth signalsHorizontal scan rate) of 15 to 240 kHz depending on the refresh rate of the CRT and the number of horizontal lines to be drawn (the vertical resolution of the CRT). The higher frequency makes it more susceptible to interference, so an automatic frequency control (AFC) circuit may be used to lock the phase of the horizontal deflection signal to that of a sync signal, to prevent the image from becoming distorted diagonally. The vertical frequency varies according to the refresh rate of the CRT. So a CRT with a 60 Hz refresh rate has a vertical deflection circuit running at 60 Hz. The horizontal and vertical deflection signals may be generated using two circuits that work differently; the horizontal deflection signal may be generated using a voltage controlled oscillator (VCO) while the vertical signal may be generated using a triggered relaxation oscillator. In many TVs, the frequencies at which the deflection coils run is in part determined by the inductance value of the coils.

Due to the high frequency at which the horizontal deflection coils operate, the energy in the deflection coils must be recycled to reduce heat dissipation. Recycling is done by transferring the energy in the deflection coils" magnetic field to a set of capacitors.

Mostly used in oscilloscopes. Deflection is carried out by applying a voltage across two pairs of plates, one for horizontal, and the other for vertical deflection. The electron beam is steered by varying the voltage difference across plates in a pair; For example, applying a voltage to the upper plate of the vertical deflection pair, while keeping the voltage in the bottom plate at 0 volts, will cause the electron beam to be deflected towards the upper part of the screen; increasing the voltage in the upper plate while keeping the bottom plate at 0 will cause the electron beam to be deflected to a higher point in the screen (will cause the beam to be deflected at a higher deflection angle). The same applies with the horizontal deflection plates. Increasing the length and proximity between plates in a pair can also increase the deflection angle.

Burn-in is when images are physically "burned" into the screen of the CRT; this occurs due to degradation of the phosphors due to prolonged electron bombardment of the phosphors, and happens when a fixed image or logo is left for too long on the screen, causing it to appear as a "ghost" image or, in severe cases, also when the CRT is off. To counter this, screensavers were used in computers to minimize burn-in.

CRTs are evacuated or exhausted (a vacuum is formed) inside an oven at approx. 375–475 °C, in a process called baking or bake-out.turbomolecular pump or a diffusion pump.getter is then fired using an RF (induction) coil. The getter is usually in the funnel or in the neck of the CRT.

CRTs used to be rebuilt; repaired or refurbished. The rebuilding process included the disassembly of the CRT, the disassembly and repair or replacement of the electron gun(s), the removal and redeposition of phosphors and aquadag, etc. Rebuilding was popular until the 1960s because CRTs were expensive and wore out quickly, making repair worth it.

Also known as rejuvenation, the goal is to temporarily restore the brightness of a worn CRT. This is often done by carefully increasing the voltage on the cathode heater and the current and voltage on the control grids of the electron gun either manually

Phosphors in CRTs emit secondary electrons due to them being inside the vacuum of the CRT. The secondary electrons are collected by the anode of the CRT.

SMPTE-C phosphors have properties defined by the SMPTE-C standard, which defines a color space of the same name. The standard prioritizes accurate color reproduction, which was made difficult by the different phosphors and color spaces used in the NTSC and PAL color systems. PAL TV sets have subjectively better color reproduction due to the use of saturated green phosphors, which have relatively long decay times that are tolerated in PAL since there is more time in PAL for phosphors to decay, due to its lower framerate. SMPTE-C phosphors were used in professional video monitors.

The phosphor coating on monochrome and color CRTs may have an aluminum coating on its rear side used to reflect light forward, provide protection against ions to prevent ion burn by negative ions on the phosphor, manage heat generated by electrons colliding against the phosphor,

Various phosphors are available depending upon the needs of the measurement or display application. The brightness, color, and persistence of the illumination depends upon the type of phosphor used on the CRT screen. Phosphors are available with persistences ranging from less than one microsecond to several seconds.

Variations in anode voltage can lead to variations in brightness in parts or all of the image, in addition to blooming, shrinkage or the image getting zoomed in or out. Lower voltages lead to blooming and zooming in, while higher voltages do the opposite.

Doming is a phenomenon found on some CRT televisions in which parts of the shadow mask become heated. In televisions that exhibit this behavior, it tends to occur in high-contrast scenes in which there is a largely dark scene with one or more localized bright spots. As the electron beam hits the shadow mask in these areas it heats unevenly. The shadow mask warps due to the heat differences, which causes the electron gun to hit the wrong colored phosphors and incorrect colors to be displayed in the affected area.

Size is limited by anode voltage, as it would require a higher dielectric strength to prevent arcing (corona discharge) and the electrical losses and ozone generation it causes, without sacrificing image brightness. The weight of the CRT, which originates from the thick glass needed to safely sustain a vacuum, imposes a practical limit on the size of a CRT.

At high deflection angles, resolutions and refresh rates (since higher resolutions and refresh rates require significantly higher frequencies to be applied to the horizontal deflection coils), the deflection yoke starts to produce large amounts of heat, due to the need to move the electron beam at a higher angle, which in turn requires exponentially larger amounts of power. As an example, to increase the deflection angle from 90 to 120°, power consumption of the yoke must also go up from 40 watts to 80 watts, and to increase it further from 120 to 150°, deflection power must again go up from 80 watts to 160 watts. This normally makes CRTs that go beyond certain deflection angles, resolutions and refresh rates impractical, since the coils would generate too much heat due to resistance caused by the skin effect, surface and eddy current losses, and/or possibly causing the glass underneath the coil to become conductive (as the electrical conductivity of glass decreases with increasing temperature). Some deflection yokes are designed to dissipate the heat that comes from their operation.

On CRTs, refresh rate depends on resolution, both of which are ultimately limited by the maximum horizontal scanning frequency of the CRT. Motion blur also depends on the decay time of the phosphors. Phosphors that decay too slowly for a given refresh rate may cause smearing or motion blur on the image. In practice, CRTs are limited to a refresh rate of 160 Hz.quantum dot LCDs (QLEDs) are available in high refresh rates (up to 144 Hz)

CRT monitors can still outperform LCD and OLED monitors in input lag, as there is no signal processing between the CRT and the display connector of the monitor, since CRT monitors often use VGA which provides an analog signal that can be fed to a CRT directly. Video cards designed for use with CRTs may have a RAMDAC to generate the analog signals needed by the CRT.multisyncing.

Picture tube CRTs have overscan, meaning the actual edges of the image are not shown; this is deliberate to allow for adjustment variations between CRT TVs, preventing the ragged edges (due to blooming) of the image from being shown on screen. The shadow mask may have grooves that reflect away the electrons that do not hit the screen due to overscan.

If the CRT is a black and white (B&W or monochrome) CRT, there is a single electron gun in the neck and the funnel is coated on the inside with aluminum that has been applied by evaporation; the aluminum is evaporated in a vacuum and allowed to condense on the inside of the CRT.ion traps, necessary to prevent ion burn on the phosphor, while also reflecting light generated by the phosphor towards the screen, managing heat and absorbing electrons providing a return path for them; previously funnels were coated on the inside with aquadag, used because it can be applied like paint;

The screen, funnel and neck are fused together into a single envelope, possibly using lead enamel seals, a hole is made in the funnel onto which the anode cap is installed and the phosphor, aquadag and aluminum are applied afterwards.

The interior aquadag or aluminum coating was the anode and served to accelerate the electrons towards the screen, collect them after hitting the screen while serving as a capacitor together with the outer aquadag coating. The screen has a single uniform phosphor coating and no shadow mask, technically having no resolution limit.

Monochrome CRTs may use ring magnets to adjust the centering of the electron beam and magnets around the deflection yoke to adjust the geometry of the image.

Color CRTs use three different phosphors which emit red, green, and blue light respectively. They are packed together in stripes (as in aperture grille designs) or clusters called "triads" (as in shadow mask CRTs).

Color CRTs have three electron guns, one for each primary color, (red, green and blue) arranged either in a straight line (in-line) or in an equilateral triangular configuration (the guns are usually constructed as a single unit).

A shadow mask tube uses a metal plate with tiny holes, typically in a delta configuration, placed so that the electron beam only illuminates the correct phosphors on the face of the tube;aperture grille of tensioned vertical wires to achieve the same result.

Trinitron CRTs were different from other color CRTs in that they had a single electron gun with three cathodes, an aperture grille which lets more electrons through, increasing image brightness (since the aperture grille does not block as many electrons), and a vertically cylindrical screen, rather than a curved screen.

The three electron guns are in the neck (except for Trinitrons) and the red, green and blue phosphors on the screen may be separated by a black grid or matrix (called black stripe by Toshiba).

Shadow masks were replaced in TVs by slot masks in the 1970s, since slot masks let more electrons through, increasing image brightness. Shadow masks may be connected electrically to the anode of the CRT.Cromaclear; Trinitron and Diamondtron use aperture grilles while Cromaclear uses a slot mask. Some shadow mask CRTs have color phosphors that are smaller in diameter than the electron beams used to light them,

Several methods were used to create the black matrix. One method coated the screen in photoresist such as dichromate-sensitized polyvinyl alcohol photoresist which was then dried and exposed; the unexposed areas were removed and the entire screen was coated in colloidal graphite to create a carbon film, and then hydrogen peroxide was used to remove the remaining photoresist alongside the carbon that was on top of it, creating holes that in turn created the black matrix. The photoresist had to be of the correct thickness to ensure sufficient adhesion to the screen, while the exposure step had to be controlled to avoid holes that were too small or large with ragged edges caused by light diffraction, ultimately limiting the maximum resolution of large color CRTs.

After the screen is coated with phosphor and aluminum and the shadow mask installed onto it the screen is bonded to the funnel using a glass frit that may contain 65 to 88% of lead oxide by weight. The lead oxide is necessary for the glass frit to have a low melting temperature. Boron oxide (III) may also present to stabilize the frit, with alumina powder as filler powder to control the thermal expansion of the frit.amyl acetate or in a polymer with an alkyl methacrylate monomer together with an organic solvent to dissolve the polymer and monomer.

Due to limitations in the dimensional precision with which CRTs can be manufactured economically, it has not been practically possible to build color CRTs in which three electron beams could be aligned to hit phosphors of respective color in acceptable coordination, solely on the basis of the geometric configuration of the electron gun axes and gun aperture positions, shadow mask apertures, etc. The shadow mask ensures that one beam will only hit spots of certain colors of phosphors, but minute variations in physical alignment of the internal parts among individual CRTs will cause variations in the exact alignment of the beams through the shadow mask, allowing some electrons from, for example, the red beam to hit, say, blue phosphors, unless some individual compensation is made for the variance among individual tubes.

Color convergence and color purity are two aspects of this single problem. Firstly, for correct color rendering it is necessary that regardless of where the beams are deflected on the screen, all three hit the same spot (and nominally pass through the same hole or slot) on the shadow mask.intaglio printed with poor registration. Poor purity causes objects on the screen to appear off-color while their edges remain sharp. Purity and convergence problems can occur at the same time, in the same or different areas of the screen or both over the whole screen, and either uniformly or to greater or lesser degrees over different parts of the screen.

The solution to the static convergence and purity problems is a set of color alignment ring magnets installed around the neck of the CRT.magnetic fields parallel to the planes of the magnets, which are perpendicular to the electron gun axes. Often, one ring has two poles, another has 4, and the remaining ring has 6 poles.vector can be fully and freely adjusted (in both direction and magnitude). By rotating a pair of magnets relative to each other, their relative field alignment can be varied, adjusting the effective field strength of the pair. (As they rotate relative to each other, each magnet"s field can be considered to have two opposing components at right angles, and these four components [two each for two magnets] form two pairs, one pair reinforcing each other and the other pair opposing and canceling each other. Rotating away from alignment, the magnets" mutually reinforcing field components decrease as they are traded for increasing opposed, mutually cancelling components.) By rotating a pair of magnets together, preserving the relative angle between them, the direction of their collective magnetic field can be varied. Overall, adjusting all of the convergence/purity magnets allows a finely tuned slight electron beam deflection or lateral offset to be applied, which compensates for minor static convergence and purity errors intrinsic to the uncalibrated tube. Once set, these magnets are usually glued in place, but normally they can be freed and readjusted in the field (e.g. by a TV repair shop) if necessary.

On some CRTs, additional fixed adjustable magnets are added for dynamic convergence or dynamic purity at specific points on the screen, typically near the corners or edges. Further adjustment of dynamic convergence and purity typically cannot be done passively, but requires active compensation circuits, one to correct convergence horizontally and another to correct it vertically. The deflection yoke contains convergence coils, a set of two per color, wound on the same core, to which the convergence signals are applied. That means 6 convergence coils in groups of 3, with 2 coils per group, with one coil for horizontal convergence correction and another for vertical convergence correction, with each group sharing a core. The groups are separated 120° from one another. Dynamic convergence is necessary because the front of the CRT and the shadow mask aren"t spherical, compensating for electron beam defocusing and astigmatism. The fact that the CRT screen isn"t spherical

The convergence signal may instead be a sawtooth signal with a slight sine wave appearance, the sine wave part is created using a capacitor in series with each deflection coil. In this case, the convergence signal is used to drive the deflection coils. The sine wave part of the signal causes the electron beam to move more slowly near the edges of the screen. The capacitors used to create the convergence signal are known as the s-capacitors. This type of convergence is necessary due to the high deflection angles and flat screens of many CRT computer monitors. The value of the s-capacitors must be chosen based on the scan rate of the CRT, so multi-syncing monitors must have different sets of s-capacitors, one for each refresh rate.

Dynamic convergence may instead be accomplished in some CRTs using only the ring magnets, magnets glued to the CRT, and by varying the position of the deflection yoke, whose position may be maintained using set screws, a clamp and rubber wedges.

Dynamic color convergence and purity are one of the main reasons why until late in their history, CRTs were long-necked (deep) and had biaxially curved faces; these geometric design characteristics are necessary for intrinsic passive dynamic color convergence and purity. Only starting around the 1990s did sophisticated active dynamic convergence compensation circuits become available that made short-necked and flat-faced CRTs workable. These active compensation circuits use the deflection yoke to finely adjust beam deflection according to the beam target location. The same techniques (and major circuit components) also make possible the adjustment of display image rotation, skew, and other complex raster geometry parameters through electronics under user control.

The guns are aligned with one another (converged) using convergence rings placed right outside the neck; there is one ring per gun. The rings have north and south poles. There are 4 sets of rings, one to adjust RGB convergence, a second to adjust Red and Blue convergence, a third to adjust vertical raster shift, and a fourth to adjust purity. The vertical raster shift adjusts the straightness of the scan line. CRTs may also employ dynamic convergence circuits, which ensure correct convergence at the edges of the CRT. Permalloy magnets may also be used to correct the convergence at the edges. Convergence is carried out with the help of a crosshatch (grid) pattern.

If the shadow mask or aperture grille becomes magnetized, its magnetic field alters the paths of the electron beams. This causes errors of "color purity" as the electrons no longer follow only their intended paths, and some will hit some phosphors of colors other than the one intended. For example, some electrons from the red beam may hit blue or green phosphors, imposing a magenta or yellow tint to parts of the image that are supposed to be pure red. (This effect is localized to a specific area of the screen if the magnetization is localized.) Therefore, it is important that the shadow mask or aperture grille not be magnetized. The earth"s magnetic field may have an effect on the color purity of the CRT.

Color CRT displays in television sets and computer monitors often have a built-in degaussing (demagnetizing) coil mounted around the perimeter of the CRT face. Upon power-up of the CRT display, the degaussing circuit produces a brief, alternating current through the coil which fades to zero over a few seconds, producing a decaying alternating magnetic field from the coil. This degaussing field is strong enough to remove shadow mask magnetization in most cases, maintaining color purity.deform (bend) the shadow mask, causing a permanent color distortion on the display which looks very similar to a magnetization effect.

Dot pitch defines the maximum resolution of the display, assuming delta-gun CRTs. In these, as the scanned resolution approaches the dot pitch resolution, moiré appears, as the detail being displayed is finer than what the shadow mask can render.

Projector CRTs were available with electrostatic and electromagnetic focusing, the latter being more expensive. Electrostatic focusing used electronics to focus the electron beam, together with focusing magnets around the neck of the CRT for fine focusing adjustments. This type of focusing degraded over time. Electromagnetic focusing was introduced in the early 1990s and included an electromagnetic focusing coil in addition to the already existing focusing magnets. Electromagnetic focusing was much more stable over the lifetime of the CRT, retaining 95% of its sharpness by the end of life of the CRT.

Beam-index tubes, also known as Uniray, Apple CRT or Indextron,Philco to create a color CRT without a shadow mask, eliminating convergence and purity problems, and allowing for shallower CRTs with higher deflection angles.

Flat CRTs are those with a flat screen. Despite having a flat screen, they may not be completely flat, especially on the inside, instead having a greatly increased curvature. A notable exception is the LG Flatron (made by LG.Philips Displays, later LP Displays) which is truly flat on the outside and inside, but has a bonded glass pane on the screen with a tensioned rim band to provide implosion protection. Such completely flat CRTs were first introduced by Zenith in 1986, and used

flat tensioned shadow masks, where the shadow mask is held under tension, providing increased resistance to blooming.TV80, and in many Sony Watchmans were flat in that they were not deep and their front screens were flat, but their electron guns were put to a side of the screen.

Radar CRTs such as the 7JP4 had a circular screen and scanned the beam from the center outwards. The screen often had two colors, often a bright short persistence color that only appeared as the beam scanned the display and a long persistence phosphor afterglow. When the beam strikes the phosphor, the phosphor brightly illuminates, and when the beam leaves, the dimmer long persistence afterglow would remain lit where the beam struck the phosphor, alongside the radar targets that were "written" by the beam, until the beam re-struck the phosphor.

In oscilloscope CRTs, electrostatic deflection is used, rather than the magnetic deflection commonly used with television and other large CRTs. The beam is deflected horizontally by applying an electric field between a pair of plates to its left and right, and vertically by applying an electric field to plates above and below. Televisions use magnetic rather than electrostatic deflection because the deflection plates obstruct the beam when the deflection angle is as large as is required for tubes that are relatively short for their size. Some Oscilloscope CRTs incorporate post deflection anodes (PDAs) that are spiral-shaped to ensure even anode potential across the CRT and operate at up to 15,000 volts. In PDA CRTs the electron beam is deflected before it is accelerated, improving sensitivity and legibility, specially when analyzing voltage pulses with short duty cycles.

When displaying fast one-shot events, the electron beam must deflect very quickly, with few electrons impinging on the screen, leading to a faint or invisible image on the display. Oscilloscope CRTs designed for very fast signals can give a brighter display by passing the electron beam through a micro-channel plate just before it reaches the screen. Through the phenomenon of secondary emiss